What Does It Mean to Be Successful in Mathematics?
Our analyses of the mathematics to be learned, our reading of the research in cognitive psychology and mathematics education, our experience as learners and teachers of mathematics, and our professional judgment have led us to adopt a composite view of successful mathematics learning. Recognizing that no term completely captures all aspects of expertise, competence, knowledge, and facility in mathematics, we have chosen mathematical proficiency to express what we think it means for anyone to learn mathematics successfully.
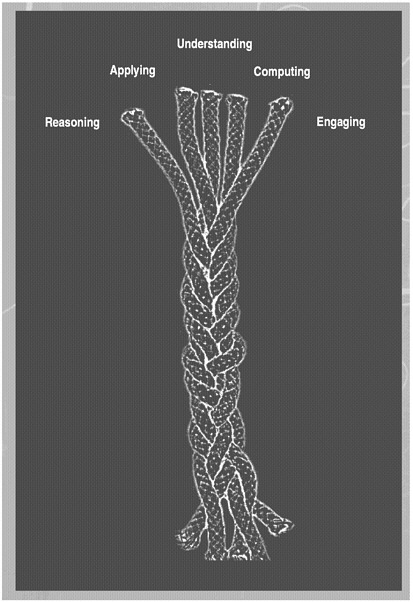
Mathematical proficiency has five strands:3
-
Understanding: Comprehending mathematical concepts, operations, and relations—knowing what mathematical symbols, diagrams, and procedures mean.
-
Computing: Carrying out mathematical procedures, such as adding, subtracting, multiplying, and dividing numbers flexibly, accurately, efficiently, and appropriately.
-
Applying: Being able to formulate problems mathematically and to devise strategies for solving them using concepts and procedures appropriately.
-
Reasoning: Using logic to explain and justify a solution to a problem or to extend from something known to something not yet known.
-
Engaging: Seeing mathematics as sensible, useful, and doable—if you work at it—and being willing to do the work.
The most important feature of mathematical proficiency is that these five strands are interwoven and interdependent. Other views of mathematics learning
have tended to emphasize only one aspect of proficiency, with the expectation that other aspects will develop as a consequence. For example, some people who have emphasized the need for students to master computations have assumed that understanding would follow. Others, focusing on students’ understanding of concepts, have assumed that skill would develop naturally. By using these five strands, we have attempted to give a more rounded portrayal of successful mathematics learning.
The overriding premise of this book is that all students can and should achieve mathematical proficiency. Just as all students can become proficient readers, all can become proficient in school mathematics. Mathematical proficiency is not something students accomplish only when they reach eighth or twelfth grade; they can be proficient regardless of their grade. Moreover, mathematical proficiency can no longer be restricted to a select few. All young Americans must learn to think mathematically if the United States is to foster the educated workforce and citizenry tomorrow’s world will demand.
The Five Strands
(1) Understanding: Comprehending mathematical concepts, operations, and relations—knowing what mathematical symbols, diagrams, procedures mean.
Understanding refers to a student’s grasp of fundamental mathematical ideas. Students with understanding know more than isolated facts and procedures. They know why a mathematical idea is important and the contexts in which it is useful. Furthermore, they are aware of many connections between mathematical ideas. In fact, the degree of students’ understanding is related to the richness and extent of the connections they have made.
For example, students who understand division of fractions not only can compute ![]() . They also can represent the operation by a diagram and make up a problem to go with the computation. (If a recipe calls for
. They also can represent the operation by a diagram and make up a problem to go with the computation. (If a recipe calls for ![]() cup of sugar and 6 cups of sugar are available, how many batches of the recipe can be made with the available sugar?)
cup of sugar and 6 cups of sugar are available, how many batches of the recipe can be made with the available sugar?)
Students who learn with understanding have less to learn because they see common patterns in superficially different situations. If they understand the general principle that the order in which two numbers are multiplied doesn’t matter—3×5 is the same as 5×3, for example—they have about half as many “number facts” to learn. Or if students understand the general principle that multiplying the dimensions of a three-dimensional object by a factor n increases its
volume by the factor n3, they can understand many situations in which objects of all shapes are proportionally expanded or shrunk. (They can understand, for example, why a 16-ounce cup that has the same shape as an 8-ounce cup is much less than twice as tall.)
Knowledge learned with understanding provides a foundation for remembering or reconstructing mathematical facts and methods, for solving new and unfamiliar problems, and for generating new knowledge. For example, students who thoroughly understand whole number operations can extend these concepts and procedures to operations involving decimals.
Understanding also helps students to avoid critical errors in problem solving—especially problems of magnitude. Any student with good number sense who multiplies 9.83 and 7.65 and gets 7,519.95 for an answer should immediately see that something is wrong. The answer can’t be more than 10 times 8 or 80, as one number is less than 10 and the other is less than 8. This reasoning should suggest to the student that the decimal point has been misplaced.
(2) Computing: Carrying out mathematical procedures, as adding, subtracting, multiplying, and dividing numbers flexibly, accurately, efficiently, and appropriately.
Computing includes being fluent with procedures for adding, subtracting, multiplying, and dividing mentally or with paper and pencil, and knowing when and how to use these procedures appropriately. Although the word computing implies an arithmetic procedure, in this document it also refers to being fluent with procedures from other branches of mathematics, such as measurement (measuring lengths), algebra (solving equations), geometry (constructing similar figures), and statistics (graphing data). Being fluent means having the skill to perform the procedure efficiently, accurately, and flexibly.
Students need to compute basic number combinations (6+7, 17−9, 8×4, and so on) rapidly and accurately. They also need to become accurate and efficient with algorithms—step-by-step procedures for adding, subtracting, multiplying, and dividing multi-digit whole numbers, fractions, and decimals, and for doing other computations. For example, all students should have an algorithm for multiplying 64 and 37 that they understand, that is reasonably efficient and general enough to be used with other two-digit numbers, and that can be extended to use with larger numbers.
The use of calculators need not threaten the development of students’ computational skills. On the contrary, calculators can enhance both understanding
|
Which side of the “math wars” is correct? Reform efforts during the 1980s and 1990s downplayed computational skill, emphasizing instead that students should understand and be able to use math. In extreme cases, students were expected to invent math with little or no assistance. Reactions to these efforts led to increased attention to memorization and computational skill, with students expected to internalize procedures presented by teachers or textbooks. The clash of these contrasting positions has been called the “math wars.” Which position is correct? Neither. Both are too narrow. When people advocate only one strand of proficiency, they lose sight of the overall goal. Such a narrow treatment of math may well be one reason for the poor performance of U.S. students in national and international assessments. Math instruction cannot be effective if it is based on extreme positions. Students become more proficient when they understand the underlying concepts of math, and they understand the concepts more easily if they are skilled at computational procedures. U.S. students need more skill and more understanding along with the ability to apply concepts to solve problems, to reason logically, and to see math as sensible, useful, and doable. Anything less leads to knowledge that is fragile, disconnected, and weak. |
and computing.4 But as with any instructional tool, calculators and computers can be used effectively or not so effectively. Teachers need to learn how to use these tools—and teach students to use them—in ways that support and integrate the strands of proficiency.
Accuracy and efficiency with procedures are important, but computing also supports understanding. By working through procedures that are general enough for solving a whole class of problems, such as a procedure for adding any two fractions, students gain appreciation for the fact that mathematics is predictable, well structured, and filled with patterns.
When students merely memorize procedures, they may fail to understand the deeper ideas that could make it easier to remember—and apply—what they learn. When they are subtracting, for example, many children subtract the smaller number from the larger in each column, no matter where it is, so wrong answers like the following are common:

Children who learn to subtract with understanding rarely make this kind of error.5
Developing computational skill and developing understanding are often seen as competing for attention in school mathematics. But pitting skill against understanding creates a false dichotomy. Understanding makes it easier to learn skills, while learning procedures can strengthen and develop mathematical understanding.
(3) Applying: Being able to formulate problems mathematically and to devise strategies for solving them using concepts and procedures appropriately.
Applying involves using one’s conceptual and procedural knowledge to solve problems. A concept or procedure is not useful unless students recognize when and where to use it—as well as when and where it does not apply. In school, students are given specific problems to solve, but outside school they encounter situations in which part of the difficulty is figuring out exactly what the problem is. Therefore, students also need to be able to pose problems, devise solution strategies, and choose the most useful strategy for solving problems. They need to know how to picture quantities in their minds or draw them on paper, and they need to know how to distinguish what is known and relevant from what is unknown.
Routine problems can always be solved using standard procedures. For example, most children in second grade know that they must add to answer the following question: “If 12 students are on the minibus and 7 more get on, how many students are on the bus?” But for nonroutine problems, students must invent a way to understand and solve the problem. For example, second graders might be asked the following question: “A minibus has 7 seats that hold 2 or 3 students each. If there are 19 students, how many must sit 2 to a seat, and how many must sit 3 to a seat?” To come up with an answer, they must invent a solution method. They need to understand the quantities in the problem and their relationships, and they must have the computing skills required to solve the problem.
(4) Reasoning: Using logic to explain justify a solution to a problem or to extend from something known to something not yet known.
Reasoning is the glue that holds mathematics together. By thinking about the logical relationships between concepts and situations, students can navigate through the elements of a problem and see how they fit together. If provided with opportunities to explore and discuss even and odd numbers, for example, fourth graders can explain why the sum of any even and any odd number will be odd.
One of the best ways for students to improve their reasoning is to explain or justify their solutions to others. Once a procedure for adding fractions has been developed, for example, students should sometimes be asked to explain and justify that procedure rather than just doing practice problems. In the process of communicating their thinking, they hone their reasoning skills.
Reasoning interacts strongly with the other strands of mathematical proficiency, especially when students are solving problems. As students reason about a problem, they can build their understanding, carry out the needed computations, apply their knowledge, explain their reasoning to others, and come to see mathematics as sensible and doable.
(5) Engaging: Seeing mathematics as sensible, useful, and doable—if you work at it—and being willing to do the work.
Engaging in mathematical activity is the key to success. Our view of mathematical proficiency goes beyond being able to understand, compute, apply, and
|
Do students still need to learn how to compute with paper and pencil now that calculators and computers are available? Yes. The widespread availability of calculators has greatly reduced the need for performing complex calculations with paper and pencil. But students need to understand what is happening in these complex calculations, and they still need to learn to perform simpler computations with pencil and paper because that helps them develop math proficiency. For example, a certain level of skill with basic number combinations is needed to understand procedures for multiplying two-digit numbers, and computational fluency is often essential in solving problems in algebra and explaining their solutions. How much instructional time should be spent on complex penciland-paper calculations is a question that will need to be continually revisited over the next decades. |
reason. It includes engagement with mathematics: Students should have a personal commitment to the idea that mathematics makes sense and that—given reasonable effort—they can learn it and use it, both in school and outside school. Students who are proficient in mathematics see it as sensible, useful, and worthwhile, and they believe that their efforts in learning it pay off; they see themselves as effective learners, doers, and users of mathematics.
Success in mathematics learning requires being positively disposed toward the subject. Students who are engaged with mathematics do not believe that there is some mysterious “math gene” that dictates success. They believe that with sufficient effort and experience they can learn. If students are to learn, do, and use mathematics effectively, they should not look at it as an arbitrary set of rules and procedures. They need to see it instead as a subject in which things fit together logically and sensibly, and they need to believe that they are capable of figuring it out.
Engaging oneself with mathematics requires frequent opportunities to make sense of it, to experience the rewards of making sense of it, and to recognize the benefits of perseverance. As students build their mathematical proficiency, they become more confident of their ability to learn mathematics and to use it. The more mathematical concepts they understand, the more sensible the whole subject becomes. In contrast, when they think mathematics needs to be learned by memorizing rather than by making sense of it, they begin to lose confidence in themselves as learners. Students who are proficient in mathematics believe that they can solve problems, develop understanding, and learn procedures through hard work, and that becoming mathematically proficient is worthwhile for them.
Integrating the Strands of Proficiency
Just as a stool cannot stand on one leg or even two, so mathematical proficiency cannot stand on one or two isolated strands. To become mathematically proficient, students need to develop all five strands throughout their elementary school and middle school years.
At any given moment during a mathematics lesson or unit, one or two strands might be emphasized. But all the strands must eventually be addressed so that the links among them are strengthened. For example, a lesson that has the main goal of developing students’ understanding of a mathematical concept might also rely on problem solving and require a number of computations. Or students might be asked to reason about a newly introduced idea rather than sim-
ply being presented with a definition and examples. In addition, throughout the school year, students should have opportunities to focus on various strands in various combinations. If teachers routinely stress just one or two strands, ignoring the others, the mathematics their students learn is likely to be incomplete and fragile.
Developing the strands of proficiency individually is much harder than learning them together. In fact, it is almost impossible to master any one of the strands in isolation. This might be why it is so hard for students to remember, for example, all the rules for computing with fractions and decimals if that is all they learn. Addressing all the strands of proficiency makes knowledge stronger, more durable, more adaptable, more useful, and more relevant.

Integrating the strands of mathematical proficiency is entirely consistent with students’ typical approaches to learning. For example, as a child gains understanding, he or she remembers computational procedures better and uses them more flexibly to solve problems. In turn, as a procedure becomes more automatic, the child can think of other aspects of a problem and can tackle new problems, which leads to new understanding. The box on page 18 provides a further description of the integration of the strands of mathematical proficiency.
U.S. school mathematics today often assumes that children must master certain skills before they can understand the procedures and apply them—as if children cannot play a tune before they have mastered all the scales. But students can figure out that there are five and a half ![]() -foot lengths of ribbon in a
-foot lengths of ribbon in a ![]() -foot strip before they are taught to “invert and multiply.” In fact, solving such problems can help students understand the invert-and-multiply procedure. They might, for example, observe that the number of
-foot strip before they are taught to “invert and multiply.” In fact, solving such problems can help students understand the invert-and-multiply procedure. They might, for example, observe that the number of ![]() -foot lengths in 1 foot is two. Hence multiplying
-foot lengths in 1 foot is two. Hence multiplying ![]() (the reciprocal of
(the reciprocal of ![]() ) will give the total number of
) will give the total number of ![]() -foot lengths in the
-foot lengths in the ![]() -foot strip.
-foot strip.
Just as a symphony cannot be heard by listening to each instrument’s part in succession, mathematical proficiency cannot be attained by learning each of the strands of proficiency in isolation. Instruction needs to take advantage of children’s natural inclination to use all five strands of mathematical proficiency. (The box on pages 19–20 gives examples of how the strands of proficiency can be integrated in learning how to solve proportions.) In this way, students understand and know how to apply procedures that they are often expected merely to memorize.
|
Integrating the Strands of Proficiency in Learning Number Combinations Learning to add, subtract, multiply, and divide with single-digit numbers has long been characterized in the United States as “learning basic facts or number combinations,” and students traditionally have been expected simply to memorize those combinations. Research has shown, however, that students actually move through a fairly well-defined sequence of solution methods when they are learning to perform operations with single-digit numbers. This deeper understanding of student learning demonstrates how the four other strands of proficiency—in addition to computing—can be strengthened through the learning of number combinations.6 Understanding Number combinations are related. Recognizing and taking advantage of these connections can make the learning of number combinations easier and less susceptible to forgetting and error. For example, students quickly learn that 5 cookies plus 4 cookies is one more than 4 cookies plus 4 cookies. Students also learn some number combinations earlier than others. For example, they often learn doubles (e.g., 6+6, 9+9) and sums to ten (e.g., 7+3) earlier than other number combinations, and they can use this knowledge to learn other number combinations (e.g., 6+7 is one more than 6+6; 8+5 can be broken down into 8+2+3). Connections between addition and subtraction and between multiplication and division can be exploited to make it easier for students to learn subtraction and division number combinations. For example, 13–8 can be thought of as the number that needs to be added to 8 to get 13. It is easier for many students to do subtraction in this building-up way, which is related to their knowledge of addition. Applying Learning number combinations can be treated as a problem-solving activity. Students use number combinations they know to generate number combinations they do not know. For example, because multiples of 5 are relatively easy to learn, a student can use his or her knowledge of 5×8 to find 6×8. It is (5×8)+8. Reasoning As students talk about how they figured out a particular number combination, they have an opportunity to explain how they did it. By explaining their solutions, they demonstrate and refine their understanding of the relevant relationships. An example might be: “I know that 4×6 is 24 because I know my fours. And 8×6 will be another four groups of six. So 8×6 is 24 plus 24, and that’s 48.” Engaging When they consider the relationships among number combinations, students see the learning of number combinations as sensible, not simply as the learning of arbitrary associations between numbers. They begin to see themselves as capable of using numbers to solve practical problems. They also learn that they can generate number combinations if they forget them. They have resources to learn on their own and do not have to depend on a teacher to tell them whether they have the right answer. |
|
Mathematical Proficiency in the Development of Proportional Reasoning Results from national assessments indicate that solving proportion problems such as “If a girl can read 3 pages in 4 minutes, at this rate how many pages will she have read in 10 minutes?” is often difficult for U.S. eighth graders. However, when students are encouraged to explore proportional situations in a variety of problem contexts, they naturally draw on all the strands of mathematical proficiency. Further, encouraging them to integrate the strands while they are learning how to solve proportions can lead to higher achievement.7 For an illustration of how students integrate the strands of proficiency, consider the work of several fifth-grade students who were given the following problem:8  Ellen, Jim, and Steve bought three helium-filled balloons priced at 3 for $2. They decided to go back to the store and buy enough balloons for everyone in the class. How much would they pay for 24 more balloons? Belinda got the correct answer ($16) by drawing 24 circles on her paper and then crossing out three and writing $2. She continued to cross out circles in groups of three, keeping track of the $2 amounts in a column, and then added the column of $2s. Damon divided 24 by 3 to arrive at $8; then he divided 24 by 2 to arrive at $12. Unable to reconcile his two answers, he resorted to using a set of cubes; he formed eight groups of three cubes each. He announced that the |
|
answer was $16, explaining that because each pack of three balloons cost $2 and there were eight packs, 2×8=16. Marti calculated the unit price for one balloon by dividing $2 by 3 on her calculator. She got 0.6667, which she called “a wacky number.” She multiplied this result by 24 to get 16. Later, Marti was telling her older brother about the different ways the children in her class had solved the balloon problem. Her brother showed her another way by using equivalent fractions: $2 for three balloons is equivalent to how much for 24 balloons? (That is, The ways in which these children solved the problem demonstrate that mastery of proportional reasoning requires integrating all the strands of proficiency. They all solved the problem, but each child used a different technique, some more sophisticated than others. They did not just randomly perform operations on the numbers, as Damon started to do. They all understood that the relationship between the balloons and the dollars must remain the same, as Belinda’s circles and column of $2s illustrate. Being fluent with various computational procedures such as counting, multiplying, and dividing helped each student attack the problem. Each child also was able to reason about the situation and to explain to the others what he or she was doing. They all expected to be able to make sense of the problem and persisted until they reached a solution they were sure of, even though Damon began with two different answers and Marti with a “wacky number.” This example illustrates how students can use their own sense-making skills to get started in a complex domain such as this. As they gain more experience with proportional situations, they can continue to develop and learn more efficient methods without losing contact with the other strands of mathematical proficiency. |
Developing Proficiency Throughout the Elementary and Middle School Years
Mathematical proficiency cannot be characterized as simply present or absent. Every important mathematical idea can be understood at many levels and in many ways. Obviously, a first grader’s understanding of addition is not the same as that of a mathematician or even an average adult. But a first grader can still be proficient with single-digit addition, as long as his or her thinking in that realm incorporates all five strands of proficiency.
Proficiency in mathematics develops over time. Thus, each year they are in school, students ought to become increasingly proficient with both old and new content. For example, third graders should be more proficient with the addition of whole numbers than they were in first grade. But in every grade, students ought to be able to demonstrate mathematical proficiency in some form.
Developing Proficiency in All Students
Historically, school mathematics policy in the United States was based on the assumption that only a select group of learners should be expected to become proficient in mathematics. That assumption is no longer tenable. Young people who are unable to think mathematically are denied many of the best opportunities that society offers, and society is denied their potential contributions.
Many adults assume that differences in mathematics performance reflect differences in innate ability, rather than differences in individual effort or opportunities to learn. These expectations profoundly underestimate what children can do. The basic principles, concepts, and skills of mathematics are within reach of all children. When parents and teachers alike believe that hard work pays off, and when mathematics is taught and learned by using all the strands of proficiency, mathematics performance improves for all students.
Careful research has demonstrated that mathematical proficiency is an obtainable goal. In a handful of schools scattered across the country, high percentages of students from all backgrounds have achieved high levels of performance in mathematics. Special interventions in some low-performing schools have produced substantial progress. More is now known about how children learn mathematics and the kinds of teaching that support progress.
Research evidence demonstrates that all but a very small number of students can learn to read proficiently. They may learn at different rates and may require different amounts and types of instructional support to learn to read well, but all can become proficient in reading.9 The same is true of learning and doing mathematics.
|
What is wrong with the old ways of teaching math? Teaching focused on one strand at a time—such as mastering computational procedures followed by problem solving—has not helped most students to achieve math proficiency. Results of major national studies dating back decades suggest that students have never been particularly successful in developing computational skills beyond whole numbers, and they have been very unsuccessful in applying the skills they have learned.10 They also haven’t demonstrated much understanding of the math concepts used in either computation or problem solving. |
|
How can teachers develop all the strands of math proficiency when they already have so much to teach? By teaching math in an integrated fashion, teachers will actually save time in the long run. They will eliminate the need to go over the same content time and again because students did not learn it well in the first place. They will not spend so much time on a single strand, deferring the other strands until students “are ready.” Rather, the five strands will support one another, which will make learning more effective and enduring. |
|
Success in Classrooms of Disadvantaged Students The evidence from research suggests that students can achieve proficiency if mathematics is taught in a coherent, integrated way. For example, a large-scale study of 150 first-grade to sixth-grade classrooms serving low-income families demonstrated the advantages of teaching for mathematical proficiency. One set of classrooms used a conventional computation-oriented curriculum. Another set stressed conceptual understanding and expanded the range of mathematical topics included in the curriculum beyond arithmetic. In the latter classrooms, teachers used multiple representations of mathematical ideas to support understanding, focused on nonroutine problems to strengthen application of concepts, emphasized multiple solutions to problems to develop computing fluency, and held classroom discussions requiring logical reasoning that explored alternative solutions or meanings of mathematical procedures or results.  Students in the latter classrooms performed substantially better than those in the conventional classrooms. At the end of the two-year study, these students not only had a greater grasp of advanced skills but also had better computational skills. Similar results were found for reading and writing. A commonly held myth in education is that students in high-poverty classrooms should not engage in academically challenging work until they have mastered the basic skills. This study dispels that myth. It shows that teaching that is organized around all the strands of mathematical proficiency is especially appropriate and effective with disadvantaged students.11 |