5
Phosphorus
BACKGROUND INFORMATION
Overview
Phosphorus is most commonly found in nature in its pentavalent form in combination with oxygen, as phosphate (PO43−). Phosphorus (as phosphate) is an essential constituent of all known protoplasm and its content is quite uniform across most plant and animal tissues. Except for specialized cells with high ribonucleic acid content, and for nervous tissue with high myelin content, tissue phosphorus occurs at concentrations ranging approximately from 0.25 to 0.65 mmol (7.8 to 20.1 mg)/g protein. A practical consequence is that, as organisms consume other organisms lower in the food chain (whether animal or plant), they automatically obtain their phosphorus.
Phosphorus makes up about 0.5 percent of the newborn infant body (Fomon and Nelson, 1993), and from 0.65 to 1.1 percent of the adult body (Aloia et al., 1984; Diem, 1970). Eighty-five percent of adult body phosphorus is in bone. The remaining 15 percent is distributed through the soft tissues (Diem, 1970). Total phosphorus concentration in whole blood is 13 mmol/liter (40 mg/dl), most of which is in the phospholipids of red blood cells and plasma lipoproteins. Approximately 1 mmol/liter (3.1 mg/dl) is present as inorganic phosphate (Pi). This inorganic phosphate component, while a tiny fraction of body phosphorus (< 0.1 percent), is of critical importance. In adults this component makes up about 15 mmol (465 mg) and is located mainly in the blood and extracellular fluid.
It is into this inorganic phosphate compartment that phosphate is inserted upon absorption from the diet and resorption from bone and from this compartment that most urinary phosphorus and hydroxyapatite mineral phosphorus are derived. This compartment is also the primary source from which the cells of all tissues derive both structural and high-energy phosphate.
Structurally, phosphorus occurs as phospholipids, which are a major component of most biological membranes, and as nucleotides and nucleic acids. The functional roles include: (1) the buffering of acid or alkali excesses, hence helping to maintain normal pH; (2) the temporary storage and transfer of the energy derived from metabolic fuels; and (3) by phosphorylation, the activation of many catalytic proteins. Since phosphate is not irreversibly consumed in these processes and can be recycled indefinitely, the actual function of dietary phosphorus is first to support tissue growth (either during individual development or through pregnancy and lactation) and, second, to replace excretory and dermal losses. In both processes it is necessary to maintain a normal level of Pi in the extracellular fluid (ECF), which would otherwise be depleted of its phosphorus by growth and excretion.
Physiology of Absorption, Metabolism, and Excretion
Food phosphorus is a mixture of inorganic and organic forms. Intestinal phosphatases hydrolyze the organic forms contained in ingested protoplasm, and thus most phosphorus absorption occurs as inorganic phosphate. On a mixed diet, net absorption of total phosphorus in various reports ranges from 55 to 70 percent in adults (Lemann, 1996; Nordin, 1989; Stanbury, 1971) and from 65 to 90 percent in infants and children (Wilkinson, 1976; Ziegler and Fomon, 1983). There is no evidence that this absorption efficiency varies with dietary intake. In the data from both Stanbury (1971) and Lemann (1996), the intercept of the regression of adult fecal phosphorus on dietary phosphorus is not significantly different from zero, and the relationship is linear out to intakes of at least 3.1 g (100 mmol)/day. This means that there is no apparent adaptive mechanism that improves phosphorus absorption at low intakes. This is in sharp contrast to calcium, for which absorption efficiency increases as dietary intake decreases (Heaney et al., 1990b) and for which adaptive mechanisms exist that improve absorption still further at habitual low intakes (Heaney et al., 1989).
A portion of phosphorus absorption is by way of a saturable, active transport facilitated by 1,25-dihydroxyvitamin D (1,25(OH)2D) (Chen et al., 1974; Cramer, 1961). However, the fact that fractional
phosphorus absorption is virtually constant across a broad range of intakes suggests that the bulk of phosphorus absorption occurs by passive, concentration-dependent processes. Also, even in the face of dangerous hyperphosphatemia, phosphorus continues to be absorbed from the diet at an efficiency only slightly lower than normal (Brickman et al., 1974).
Phosphorus absorption is reduced by ingestion of aluminum-containing antacids and by pharmacologic doses of calcium carbonate. There is, however, no significant interference with phosphorus absorption by calcium at intakes within the typical adult range.
Excretion of endogenous phosphorus is mainly through the kidneys. Inorganic serum phosphate is filtered at the glomerulus and reabsorbed in the proximal tubule. The transport capacity of the proximal tubule for phosphorus is limited; it cannot exceed a certain number of mmol per unit time. This limit is called the tubular maximum for phosphate (TmP). TmP varies inversely with parathyroid hormone (PTH) concentration; PTH thereby adjusts renal clearance of Pi. At filtered loads less than the TmP (for example, at low plasma Pi values), most or all of the filtered load is reabsorbed, and thus plasma phosphate levels can be at least partially maintained. By contrast, at filtered loads above the TmP, urinary phosphorus is a linear function of plasma phosphate (Bijvoet, 1969; Lemann, 1996; Nordin, 1989). In the healthy adult, urine phosphorus is essentially equal to absorbed diet phosphorus, less small amounts of phosphorus lost in shed cells of skin and intestinal mucosa.
This regulation of phosphorus excretion is apparent from early infancy. In infants, as in adults, the major site of regulation of phosphorus retention is at the kidney. In studies of infants receiving different calcium intakes (DeVizia et al., 1985; Moya et al., 1992; Williams et al., 1970; Ziegler and Fomon, 1983), phosphorus retention did not differ even with high amounts of dietary calcium (calcium:phosphorus [Ca:P] molar ratios of 0:6, 1:1, or 1.4:1). Any reduction in absorption of phosphorus due to high amounts of dietary calcium were compensated for by parallel reductions in renal phosphorus excretion (DeVizia et al., 1985; Fomon and Nelson, 1993; Moya et al., 1992). The least renal excretory work to maintain normal phosphorus homeostasis would be achieved with human milk as the major source of minerals during the first year of life.
Regulation of the Serum Inorganic Phosphate Concentration
Pi levels are only loosely regulated. Normal Pi levels decline with age from infancy to maturity (Table 5-1). The most likely reason for the
TABLE 5-1 Normative Values for Serum Inorganic Phosphorus (mmol/liter) for Age
|
Age (y) |
Mean |
2.5 Percentile |
97.5 Percentile |
|
0–0.5 |
2.15 |
1.88 |
2.42 |
|
2 |
1.81 |
1.43 |
2.20 |
|
4 |
1.77 |
1.38 |
2.15 |
|
6 |
1.72 |
1.33 |
2.11 |
|
8 |
1.67 |
1.29 |
2.06 |
|
10 |
1.63 |
1.24 |
2.01 |
|
12 |
1.58 |
1.19 |
1.97 |
|
14 |
1.53 |
1.15 |
1.92 |
|
16 |
1.49 |
1.10 |
1.88 |
|
20 |
1.39 |
1.01 |
1.78 |
|
Adult |
1.15 |
0.87 |
1.41 |
higher Pi in newborn infants than in older children and adults is the lower glomerular filtration rate (GFR) of infants. GFR is about 32 ml/min/1.73 m2 at about 1 week of age, and rises to 87 at 4 to 6 months (Brodehl et al., 1982; Svenningsen and Lindquist, 1974). In the first months of life, plasma Pi concentration appears to be a reflection both of renal glomerular maturity and of the amount of dietary intake. Mean serum Pi appears to decline by about 0.3 mmol/liter (0.9 mg/dl) across the second half of the first year of life (Specker et al., 1986). Human milk-fed, compared with formula-fed, infants have a slightly lower plasma Pi (2.07 versus 2.25 mmol/liter or 6.4 versus 7.0 mg/dl) which is simply a function of differences in intake (Greer et al., 1982c; Specker et al., 1986); Gaucasian compared with African American infants have a slightly higher plasma Pi irrespective of type of milk feeding (Specker et al., 1986).
The general relationship between absorbed phosphorus intake and plasma Pi in adults is set forth in Figure 5-1, derived by Nordin (1989) from the infusion studies of Bijvoet (1969). (In Bijovet's studies, a neutral phosphate solution was infused intravenously at a steadily increasing rate and produced a controlled hyperphosphatemia.) The achieved plasma Pi could thus be directly related to the quantity entering the circulation. Plasma Pi rises rapidly at low intakes, since the filtered load will be below the TmP, and little of the absorbed phosphorus will be lost in the urine (Figure 5-1). The steep, ascending portion of the curve thus represents a filling up of the extracellular fluid space with absorbed phosphate. At higher intakes, urinary excretion rises to match absorbed input and plasma levels change much more slowly.
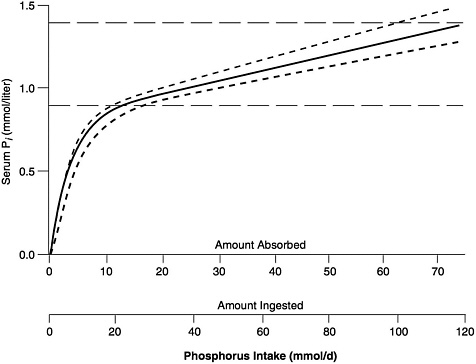
FIGURE 5-1 Relation of serum Pi to absorbed intake in adults with normal renal function. (See Nordin [1989] for further details.) The solid curve can be empirically approximated by the following equation: Pi = 0.00765 × AbsP + 0.8194 × (1 − e(−0.2635 × AbsP), in which Pi = serum Pi (in mmol/liter), and AbsP = absorbed phosphorus intake (also in mmol). Solving this equation for the lower and upper limits of the normal range for Pi, as well as for its midpoint, yields the values presented in Table 5-1. The dashed horizontal lines represent approximate upper and lower limits of the normal range, while the dashed curves reflect the relationship between serum Pi and ingested intake for absorption efficiencies about 15 percent higher and lower than average. (© Robert P. Heaney, 1996. Reproduced with permission.)
The relationship shown in Figure 5-1 holds only in adult individuals with adequate renal function; that is, the slow rise of plasma Pi with rising phosphorus intake over most of the intake range applies only so long as excess absorbed phosphate can be spilled into the urine. However, in individuals with reduced renal function, phosphorus clearance remains essentially normal so long as GFR is at least 20 percent of mean adult normal values, largely because tubular reabsorption is reduced to match the reduction in filtered load. Below that level, excretion of absorbed phosphate requires higher and higher levels of plasma Pi to maintain a filtered load at least
equal to the absorbed load. This is the reason for the hyperphosphatemia typically found in patients with end-stage renal disease.
Another process depleting the blood of Pi is mineralization of nucleated bone and cartilage matrix. The amorphous calcium phosphate formed in the first stages of mineralization exhibits a Ca:P molar ratio of about 1.33:1, or very close to the molar ratio of Ca:P in adult ECF. While outside of a mineralizing environment, ECF calcium and phosphorus concentrations are indefinitely stable at physiological pH and pCO2, ECF is supersaturated in the presence of the hydroxyapatite crystal lattice. Hence, ECF supports calcium phosphate deposition only in the presence of a suitable crystal nucleus. As a consequence, in nonosseous tissues, ECF [Ca2+] and Pi concentrations will be essentially what can be measured in peripheral venous blood. However, at active bone-forming sites, ECF is depleted of both its calcium and its phosphate. Osteoblast function seems not to be appreciably affected by ECF [Ca2+], but like other tissues, the osteoblast needs a critical level of Pi in its bathing fluid for fully normal cellular functioning. Local Pi depletion both impairs osteoblast function and limits mineral deposition in previously deposited matrix. Finally, it should be noted that ECF Pi levels are indirectly supported by two mechanisms that amount to a weak, negative feedback type of control. One occurs via release of phosphate from bone, and the other via regulation of the renal 1-α hydroxylase. Still Pi concentration affects the responsiveness of the osteoclast to PTH: for any given PTH level, resorption is higher when Pi levels are low, and vice versa (Raisz and Niemann, 1969). High Pi values, by reducing bony responsiveness to PTH, lead to increased PTH secretion in order to maintain calcium homeostasis, and thereby to a lowering of ECF Pi. Similarly, high plasma Pi levels suppress renal synthesis of 1,25(OH)2D (Portale et al., 1989), thereby slightly reducing net phosphorus absorption from the diet. Both mechanisms reduce phosphorus input into the ECF when Pi is high and augment it when Pi is low. However, in neither circumstance is the effect on plasma Pi more than modest. (See below for other special circumstances.)
Factors Affecting the Phosphorus Requirement
Bioavailability
Most food sources exhibit good phosphorus bioavailability. There is, however, one major exception. All plant seeds (beans, peas, cereals, nuts) contain a nonprotoplasmic, storage form of phosphate,
phytic acid. The digestive systems of most mammals cannot hydrolyze phytic acid, and hence its phosphorus is not directly available. However, some foods contain phytase, as do some colonic bacteria. Thus, a variable amount of phytate phosphorus becomes available. However, in gnotobiotic animals, no phytate phosphorus is absorbed (Wise and Gilburt, 1982). In at least one study (Parfitt et al., 1964), up to 50 percent of phytate phosphorus was absorbed, representing the combined effect of food phytases and bacterial enzymes. Also, yeasts can hydrolyze phytate, and thus whole grain cereals incorporated into leavened bread products have higher phosphate bioavailability than cereal grains used, for example, in unleavened bread or breakfast cereals. Finally, unabsorbed calcium in the digestate complexes with phytic acid and interferes with bacterial hydrolysis of phytate (Sandberg et al., 1993; Wise and Gilburt, 1982). This may be a part of the explanation for calcium's interference with phosphorus absorption.
In infants, both the quantity of ingested phosphorus and the dietary bioavailability vary by type of milk fed. The efficiency of absorption is highest from human milk (85 to 90 percent) (Williams et al., 1970), intermediate from cow milk (72 percent) (Williams et al., 1970; Ziegler and Fomon, 1983), and lowest from soy formulas, which contain phytic acid (~59 percent) (Ziegler and Fomon, 1983). Because infant formulas contain substantially greater amounts of phosphorus than human milk, the absorbed phosphorus from cow milk and soy formulas is twice that attained by human milk-fed infants (Moya et al., 1992). The higher amounts of phosphorus (and also calcium and other mineral elements) in formulas that are based on cow milk or soy isolate protein effectively offset the lower mineral absorption of these formulas relative to human milk.
Relatively low intakes of phosphorus, as occur with human milk, may actually confer an advantage to the infant by virtue of the low residual phosphorus in the lower bowel. Low intestinal phosphorus concentrations lower the fecal pH (Manz, 1992), which in turn may reduce proliferation of potentially pathogenic microorganisms and provide an immune protective effect.
Nutrient-Nutrient Interactions
Calcium. In the past, considerable emphasis was placed on the Ca:P ratio of the diet (for example, Chinn, 1981), particularly in infant nutrition (for example, Fomon and Nelson, 1993). The concept has some utility under conditions of rapid growth (in which a
large share of the ingested nutrients is converted into tissue mass), but it has no demonstrable relevance in adults. An optimal ratio ensures that, if intake of one nutrient is adequate for growth, the intake of the associated nutrient will also be adequate without a wasteful surplus of one or the other. However, the ratio by itself is of severely limited value, in that there is little merit to having the ratio “correct” if the absolute quantities of both nutrients are insufficient to support optimal growth.
Furthermore, the intake ratio, by itself, fails to take into account both differing bioavailabilities and physiological adaptive responses. For example, in term-born infants during the first year of life, a higher calcium content of soy-based formulas was found to reduce phosphorus absorption, but phosphorus retention was similar because of offsetting changes in renal phosphorus output (DeVizia et al., 1985). Substitution of lactose with sucrose and/or hydrolyzed corn syrup solids had no effect on the efficiency of phosphorus absorption from formulas based on cow milk (Moya et al., 1992) or soy protein (Ziegler and Fomon, 1983).
Estimates of optimal Ca:P intake ratios have frequently been based on the calcium and phosphorus needs of bone building. The molar ratio of Ca:P in synthetic hydroxyapatite is 1.67:1; in actual bone mineral, usually closer to 1.5:1; and in amorphous calcium-phosphate (the first mineral deposited at the mineralizing site), 1.3:1 (Nordin, 1976). However, during growth, soft tissue will be accreting phosphorus as well. On average, lean soft tissue growth accounts for about 1 mmol (31 mg) phosphorus for every 5 mmol (155 mg) added to bone (Diem, 1970). Since soft tissue accretion of calcium is negligible compared with skeletal calcium accretion, an absorbed Ca:P molar ratio sufficient to support the sum of bony and soft tissue growth would be ~1.3:1 (assuming equivalent degrees of renal conservation of both nutrients). The corresponding ingested intake ratio must consider the differing absorption efficiencies for dietary calcium and phosphorus. In infants, with net absorptions for calcium and phosphorus of approximately 60 and 80 percent respectively, the intake ratio matching tissue accretion would be ~2:1 (see also the section “Birth through 12 Months, ” below). This value is somewhat higher than the Ca:P molar ratio of human milk, which in most populations is in the range of 1.5:1 (Fomon and Nelson, 1993).
Human milk must be presumed to be optimal for the infant's nutritional needs. The disparity between its ratio of ~1.5:1 and the ingested ratio calculated above reflects both the uncertainties in
the estimates on which the calculation is based and the limitations inherent in using such calculations.
If growth were the only consideration, the intake ratio would have to be substantially higher than 2:1 after infancy, because calcium absorption drops more sharply with age than does phosphorus absorption (Abrams et al., 1997b; Fomon and Nelson, 1993). However, as larger fractions of ingested food are used for energy (and a correspondingly smaller proportion for growth), the notion of a dietary Ca:P molar ratio has little meaning or value, particularly since, on a mixed diet, there is likely to be a relative surplus of phosphorus. Under such circumstances it would be inappropriate to conclude, simply on the basis of a departure from some theoretical Ca:P ratio, either that calcium intake should be elevated or, phosphorus intake reduced. In balance studies in human adults, Ca:P molar ratios ranging from 0.08:1 to 2.40:1 (a 30-fold range) had no effect on either calcium balance or calcium absorption (Heaney and Recker, 1982; Spencer et al., 1965, 1978a). Thus, for the reasons cited, there is little or no evidence for relating the two nutrients, one to the other, during most of human life.
Intake of Phosphorus
The USDA Continuing Survey of Food Intake of Individuals (CSFII) in 1994, adjusted by the method of Nusser et al. (1996), indicated that the mean daily phosphorus intake from food in males aged 9 and over was 1,495 mg (48.2 mmol) (fifth percentile = 874 mg [28.2 mmol]; fiftieth percentile = 1,445 mg [46.6 mmol]; ninety-fifth percentile = 2,282 mg [73.6 mmol]) (see Appendix D for data tables). The mean daily intake in females aged 9 and over was 1,024 mg (33 mmol) (fifth percentile = 620 mg [20 mmol]; fiftieth percentile = 1,001 mg [32.3 mmol]; ninety-fifth percentile = 1,510 mg [48.7 mmol]). In both sexes, intakes decreased at ages 51 and over. The NHANES III data show similar median intake values (Alaimo et al., 1994). National survey data for Canada are not available.
Both extent of usage of phosphate salts as additives and the amount per serving have increased substantially over the past 20 years, and the nutrient databases may not reflect these changes (Calvo and Park, 1996). For that reason, phosphorus intake may be underestimated for certain individuals who rely heavily on processed foods. However, one comparison of calculated intakes with analyzed intake data from the U.S. Food and Drug Administration's Total Diet Study found slight overestimates of phosphorus intake (by an
average of 61 mg [2 mmol]/day or 5 percent of the total intake) when intakes were calculated using USDA's nutrient database (Pennington and Wilson, 1990).
Because of the uncertainty about phosphorus values for processed foods in nutrient databases, trends in phosphorus intake may be difficult to ascertain. Daily intakes of women aged 19 to 50 years from USDA 's national surveys averaged 965 mg (31.1 mmol) in 1977, 1,039 mg (33.5 mmol) in 1985, and 1,022 mg (33.0 mmol) in 1994 (Cleveland et al., 1996; USDA, 1985). Thus, it appears that intakes from foods increased about 8 percent between 1977 and 1985, but then decreased slightly between 1985 and 1994. Food supply data show a larger increase in phosphorus consumption: 12 percent from 1980 through 1994 (from 1,480 to 1,680 mg [47.7 to 54.2 mmol]/day per capita) (USDA, 1997). However, disappearance data may be unreliable for detecting trends because phosphate additives (such as those in cola beverages) are not included. Disappearance data on phosphorus-containing additives show that the use of these additives has increased by 17 percent over the last decade (Calvo, 1993). These figures also do not reflect actual consumption, because not all phosphates included in disappearance data are actually consumed, (for example, blends of sodium tripolyphosphate and sodium hexametaphosphate are used in brines for curing meat, but the brine is rinsed off and not consumed). Nevertheless, taken together, these data suggest a substantial increase in phosphorus consumption, in the range of 10 to 15 percent, over the past 20 years.
Food Sources of Phosphorus
Phosphates are found in foods as naturally occurring components of biological molecules and as food additives in the form of various phosphate salts. These salts are used in processed foods for nonnutrient functions, such as moisture retention, smoothness, and binding.
In infants, dietary intake of phosphorus spans a wide range, depending on whether the food is human milk, cow milk, adapted cow milk formula, or soy formula (see Table 5-2). Moreover, the phosphorus concentration of human milk declines with progressing lactation, especially between 4 and 25 weeks of lactation (Atkinson et al., 1995). By contrast, more of the variation in dietary intake of phosphorus in adults is due to differences in total food intake and less to differences in food composition. Phosphorus contents of adult diets average about 62 mg (2 mmol)/100 kcal in both sexes (Carroll et al., 1983), and phosphorus:energy ratios exhibit a coefficient of variation of only
TABLE 5-2 Average Phosphorus Content and Calcium:Phosphorus Molar Ratio of Various Infant Feedings
|
Feeding Type |
P (mmol/liter) |
Ca:P Molar Ratio |
|
|
Human milka |
|||
|
1 week |
5.1 ± 0.9 |
1.3:1 |
|
|
4 weeks |
4.8 ± 0.8 |
1.4:1 |
|
|
16 weeks |
3.9 ± 0.5 |
1.5:1 |
|
|
Cows' milk formula |
12 |
1.0:1 |
|
|
Soy formulab |
15 |
1.2:1 |
|
|
Whole cows' milk |
30 |
1.0:1 |
|
about one-third that of total phosphorus intake. Nevertheless, individuals with high dairy product intakes will have diets with higher phosphorus density values, since the phosphorus density of cow milk is higher than that of most other foods in a typical diet. The same is true for diets high in colas and a few other soft drinks that use phosphoric acid as the acidulant. A 12-ounce serving of such beverages contains about 50 mg (< 2 mmol), which is only 5 percent of the typical intake of an adult woman. However, when consumed in a quantity of five or more servings per day, such beverages may contribute substantially to total phosphorus intake.
a Milk phosphorus at three different weeks of lactation (Atkinson et al., 1995).
b Phosphorus content of soy formula includes about 3 mmol/L, present as phytate phosphorus which is likely not to be bioavailable (DeVizia and Mansi, 1992).
Intake from Supplements
Phosphorus supplements are not widely used in the United States. Based on a national survey in 1986, about 10 percent of U.S. adults and 6 percent of children aged 2 to 6 years took supplements containing phosphorus (Moss et al., 1989). Usage by men and women was similar, as was the dose taken by users: a median of about 120 mg (3.9 mmol)/day and a ninety-fifth percentile of 448 mg (14.5 mmol)/day. Young children who took supplements had a median supplemental intake of only 48 mg (1.5 mmol)/day and a ninety-fifth percentile of intake of 200 mg (6.5 mmol)/day.
Effects of Inadequate Phosphorus Intake
Hypophosphatemia and Phosphorus Depletion
Inadequate phosphorus intake is expressed as hypophosphatemia.
Only limited quantities of phosphate are stored within cells, and most tissues depend upon ECF Pi for their metabolic phosphate. When ECF Pi levels are low, cellular dysfunction follows. At a whole organism level, the effects of hypophosphatemia include anorexia, anemia, muscle weakness, bone pain, rickets and osteomalacia, general debility, increased susceptibility to infection, paresthesias, ataxia, confusion, and even death (Lotz et al., 1968). The muscle weakness, which involves especially proximal muscle groups when prolonged or severe, can lead to muscle fiber degeneration. The skeleton will exhibit either rickets in children or osteomalacia in adults. In both, the disorder consists of a failure to mineralize forming growth plate cartilage or bone matrix, together with impairment of chondroblast and osteoblast function (Lotz et al., 1968). These severe manifestations are usually confined to situations in which ECF Pi falls below ~0.3 mmol/liter (0.9 mg/dl).
Phosphorus is so ubiquitous in various foods that near total starvation is required to produce dietary phosphorus deficiency. However, movement of sugar into cells pulls Pi into the cells as well. Refeeding of energy-depleted individuals, either orally or parenterally, without adequate attention to supplying Pi, can precipitate extreme, even fatal, hypophosphatemia (Bushe, 1986; Dale et al., 1986; Knochel, 1977, 1985; Ritz, 1982; Silvis and Paragas, 1972; Stein et al., 1966; Travis et al., 1971; Young et al., 1985). Such outcomes can occur on recovery from alcoholic bouts, from diabetic ketoacidosis, and on refeeding with calorie-rich sources without paying attention to phosphorus needs. Also, aluminum-containing antacids, by binding diet phosphorus in the gut, can, when consumed in high doses, produce hypophosphatemia in their own right, as well as aggravate phosphate deficiency related to other problems (Lotz et al., 1968).
In full-term infants, severe hypophosphatemia from purely dietary causes is virtually unknown. It is likely to occur only in situations of poorly managed parenteral nutrition (in which intakes of phosphate are inadequate), with inappropriate administration of fluid and electrolyte therapy (which causes excessive renal phosphorus loss), or with rapid refeeding after prolonged dietary restriction (Koo and Tsang, 1997; Weinsier and Krumdieck, 1981). In the case of severely malnourished infants, especially with accompanying severe diarrhea, hypophosphatemia has been reported with an associated hypokalemia and hypotonia (Freiman et al., 1982).
ESTIMATING REQUIREMENTS FOR PHOSPHORUS
Selection of Indicators for Estimating the Phosphorus Requirement
In the past, indicators have not generally been used for the phosphorus requirement. Instead, phosphorus recommendations have been tied to calcium, usually on an equimass or equimolar basis. As noted above, that approach is unsatisfactory. The two indicators that will be considered here for the Estimated Average Requirement (EAR) are phosphorus balance and serum Pi.
Phosphorus Balance
Although phosphorus balance might seem to be a logical indicator of nutritional adequacy, it is not an adequate criterion, since an adult can be in zero balance at an intake inadequate to maintain serum Pi within the normal range. Even during growth, balance will be positive in direct proportion to soft tissue and bony accumulation, but so long as plasma Pi is high enough, the degree of positive balance will be limited either by the genetic programming or by availability of other nutrients. Furthermore, the balance data that would be needed to bracket the requirement during growth are not available. And during senescence, if there is loss of bone or soft tissue mass, phosphorus balance will be negative. However, so long as plasma Pi remains within normal limits, these balances will reflect other changes occurring in the body and will not be an indicator of the adequacy of dietary phosphorus.
Serum Pi
Because phosphorus intake directly affects serum Pi, and because both hypo- and hyperphosphatemia directly result in dysfunction or disease, the most logical indicator of nutritional adequacy of phosphorus intake is serum Pi. If serum Pi is above the lower limits of normal for age, phosphorus intake may be considered adequate to meet cellular and bone formation needs of healthy individuals. The relationship between Pi and phosphorus intake has, however, been clearly established only for adults (see “Regulation of the Serum Inorganic Phosphate Concentration”), and while the adverse effects of low serum Pi are well understood during growth, it is harder to define the critical values for phosphorus intake associated with the normal range of Pi values in infants and children. For that reason, estimates of
requirements will be based on a factorial approach in infants, children, and adolescents and on serum Pi in adults.
There is a clear and absolute requirement for phosphorus during growth. At each growth stage, average net daily additions of bone and soft tissue mass can be approximated. Thus, the absorbed phosphorus intake needed to support such tissue accumulation can be readily estimated (see below for each relevant physiological state). In the mature adult, the requirement can be defined instead simply as the intake needed to maintain the plasma Pi within the normal range. As with all continuous clinical variables, there is a gray zone between the empirical normal range and values associated with evident deficiency disease. Thus, the lower end of the established normal range for serum Pi is 0.8 to 0.9 mmol/liter (2.5 to 2.8 mg/dl). Clear evidence of bony or soft tissue dysfunction are not common until serum Pi levels drop below 0.3 to 0.5 mmol/liter (0.9 to 1.6 mg/dl).
Although it is not likely that all levels of Pi within the normal range are equally salubrious for cell functioning, insufficient information exists to allow selection of any one value within the population normal range as superior to any other. The fact that growth and epiphyseal cartilage maturation in children are abnormal at even adult normal levels of Pi supports the assumption that subnormal Pi values are not adequate to sustain optimal tissue function. Therefore, in what follows, the requirement will be based on the intake associated with maintenance of serum Pi at the bottom end of the normal range. A limitation of this approach lies in the fact that available data on Pi apply mostly to the fasting state, whereas it is the integrated, 24-hour Pi that is most closely related to absorbed phosphorus intake and that constitutes the actual exposure that the tissues experience. Moreover, fasting serum Pi is only weakly correlated with current phosphorus intake (for example, Portale et al., 1987).
The approach is to define, as well as the available evidence will permit, the lower limit of normal for serum Pi in healthy individuals of various ages (Table 5-1). The exclusion of formula-fed babies from the infant data is essential since some formulas, and cow milk especially, have higher phosphorus levels than required, and hence they result in higher values for serum Pi. This fact precludes their use in establishing normative data for the requirement.
FINDINGS BY LIFE STAGE AND GENDER GROUP
Birth through 12 Months
There are no functional criteria for phosphorus status that reflect response to dietary intake in infants. Thus recommended intakes of phosphorus are based on Adequate Intakes (AIs) that reflect observed mean intakes of infants fed principally with human milk.
Indicators Used to Set the AI
Human Milk. Human milk is recognized as the optimal source of milk for infants throughout at least the first year of life and as a sole nutritional source for infants during the first 4 to 6 months of life (IOM, 1991). Furthermore, there are no reports of exclusively human milk-fed, vitamin D-replete, full-term infants manifesting any evidence of phosphorus deficiency. Therefore, consideration of the AI for phosphorus in infants is based on data from term-born healthy infants fed human milk as the principal fluid milk during the first year of life. The approach was to set the AI at the mean of usual intakes of phosphorus as derived from studies where intake of human milk was measured using test weighing, and intake of food was determined by dietary records for 3 days or more. The following limited data on infants were also reviewed and considered as supportive evidence for the derived AIs.
Serum Pi. Using the term-born infant fed human milk as the model, the target range for serum Pi (the most appropriate biochemical indicator of dietary phosphorus adequacy during early life), is 2.42 to 1.88 mmol/liter (7.5 to 5.8 mg/dl). This wide range is a function of the precipitous fall in serum Pi during the first 6 weeks of life (Greer et al., 1982b). The reasons for the well-documented decline in serum Pi in infants are: (1) an increase in GFR as the infant's kidneys mature postnatally (McCrory et al., 1950), (2) a decline in phosphorus concentration in breast milk with advancing lactation (Table 5-2), and (3) possibly an inappropriate PTH response to rising serum Pi during early neonatal life (DeVizia and Mansi, 1992). However, there is evidence that the parathyroid glands can respond in early life since high phosphorus feedings (Ca:P molar ratio 1:2.5) resulted in serum PTH, which was significantly higher than in breast-fed infants at 2, 7, and 14 days of age
(Janas et al., 1988). Similarly, in infants 4 to 6 months of age, the feeding of infant cereal in addition to standard infant formula, compared with formula alone, produced a significantly higher serum PTH in young infants. This result is often attributed to the dietary phosphorus load from the cereal (Bainbridge et al., 1996), but probably it is due to phytate complexation of food calcium with consequent reduction in calcium absorption.
Balance. Mean phosphorus intakes from human milk of 102 mg (3.3 mmol)/day in healthy infants (n = 33) supported a positive phosphorus balance; an efficiency of absorption of 85 percent provided a net retention of 59 mg (1.9 mmol)/day (Fomon and Nelson, 1993). In considering the possible functional indicators, positive rates of bone mineral accretion have been observed in human milk-fed infants over the first 6 months of life (Hillman et al., 1988; Mimouni et al., 1993; Specker et al., 1997). Although the absolute amount of bone mineral accrued over the first 6 months (measured both for the humerus alone and for the whole body) was less in infants fed human milk than in those fed modified formulas (Hillman et al., 1988; Specker et al., 1997), this difference does not have known long-term consequences. By 12 months of age whole body mineral content was similar among groups irrespective of feeding in the first 6 months (Specker et al., 1997). Perhaps the slower initial accretion of bone demonstrated in human milk-fed infants represents the physiological norm.
Accretion. Increments of body content of phosphorus over the first 6 months of life are estimated to range from 11 to 28 mg (0.35 to 0.9 mmol)/day (Fomon and Nelson, 1993); amounts that are easily obtained from human milk (Fomon and Nelson, 1993; Williams et al., 1970). Increments in whole body phosphorus between 6 and 12 months are calculated to be about 31 mg (1 mmol)/day. Assuming an efficiency of absorption of 52 percent (from high-calcium, soy-based formula) (DeVizia et al., 1985) or higher, intakes above 90 mg (2.9 mmol) are probably in excess of functional needs. Unfortunately no information exists on response of infants fed varying phosphorus intakes in the second 6 months of life.
Differences in phosphorus needs between infants fed human milk and those fed infant formula are considered in the “Special Considerations ” section.
AI Summary: Ages 0 through 6 months
The estimate of the AI is based on two studies, Allen et al. (1991) and Butte et al. (1984), which reported mean intake of human milk of 780 ml/day based on test weighing of term-born infants, and a reported average phosphorus concentration in human milk after the first month of lactation of 124 mg/liter (4 mmol/liter) (Atkinson et al., 1995) (Table 5-2). Using the mean value for intake of human milk (780 ml/day), the AI would be 100 mg (3.2 mmol)/day for infants 0 through 6 months of age. Based on the available data on urinary and serum Pi, this phosphorus intake obtained from human milk over the first 6 months of life results in a circulating Pi concentration that does not require excessive renal excretion of phosphorus.
AI Summary: Ages 7 through 12 months
The basis of the AI for this age group is the average intake of phosphorus from human milk plus that obtained from infant foods. For infants over 6 months of age, there are no available data on dietary phosphorus intakes from the combination of human milk and solid foods. Thus, values for the phosphorus intake from solid food were derived from data on formula-fed infants, assuming that infants who are fed human milk have similar intakes of solid food during this time.
Based on the data from Dewey et al. (1984), mean milk intake by infants was 600 ml/day, and assuming a milk phosphorus concentration of 124 mg/liter (4 mmol/liter), the average phosphorus intake from human milk alone would be 75 mg (2.4 mmol)/day. To determine total phosphorus intake during the ages 7 through 12 months, an estimate of the contribution of solid foods must be made. Using the recent data of Specker et al. (1997), 40 infants fed standard formula and solid food had mean phosphorus intake from solid food of 151 mg (4.9 mmol) and 255 mg (8.2 mmol)/day at 9 and 12 months, respectively. This finding is comparable to the dietary phosphorus from solid foods estimated to be 155 to 186 mg (5 to 6 mmol)/day based on data from 24-hour dietary intakes from the 1976–1980 NHANES II for infants aged 7 to 12 months (Montalto and Benson, 1986).
Based on the literature cited above, the mean daily total phosphorus intake from human milk (75 mg [2.4 mmol] ) and solid foods (200 mg [6.5 mmol]) is 275 mg (8.9 mmol) between the ages 7 and 12 months. The data are averaged for solid food intake based on the above observations of infants. Thus, the AI for infants ages 7 through 12 months is
set at 275 mg (8.9 mmol)/day, which falls between the fifth and tenth percentiles for phosphorus intake of infants 7 to 11 months of age from the 1994 CSFII intake data. However, it must be emphasized that this database specifically excluded infants fed human milk, and thus the formula or cow milk in the infants' diets would elevate the population distribution and mean phosphorus intakes.
|
AI for Infants |
0 through 6 months |
100 mg (3.2 mmol)/day |
|
7 through 12 months |
275 mg (8.9 mmol)/day |
Special Considerations
Infant Formula. Much of the available data on phosphorus intake in infancy, particularly during the second 6 months of life, were collected prior to the 1980s when infants were often fed diluted evaporated milk formulas and/or undiluted cow milk, in addition to infant cereals and solid foods. These foods were introduced at an earlier age than is the general practice in the 1990s. If cow milk were fed instead of human milk, the phosphorus intake from milk and solid foods would be about 2.5-fold higher (Montalto and Benson, 1986). Although dietary phosphorus is generally well absorbed (72 percent from whole cow milk versus 85 percent from human milk [Fomon and Nelson, 1993]), there is evidence that dietary phosphorus intake above 310 mg (10 mmol) results in a linear increase in fecal excretion.
Several studies have reported significantly lower values for serum Pi in infants fed human milk versus modified cow milk-based formulas; this finding is logical since formulas provide about three times the amount of dietary phosphorus as human milk (Fomon and Nelson, 1993; Janas et al., 1988; Lealman et al., 1976; Specker et al., 1986). However, the mean serum Pi in the formula-fed infants fell within the range observed for human milk-fed infants within the first 6 months of life. Thus, the higher intakes from modified formulas are not likely to be of physiological significance. With maturation of the renal phosphate excretion mechanism sometime after 6 weeks of life (Brodehl et al., 1982), infants should be able to adapt to a wide range of phosphorus intakes. Mean phosphorus intake from a mixed diet of formula or cow milk and solid foods ranged between 490 and 800 mg (16 and 26 mmol)/day for infants 9 to 12 months of age (Specker et al., 1997). Since in this study there were no significant differences in weight, length, or bone mineral content between infant groups, the higher intakes conferred no benefit.
Ages 1 through 3 Years
Indicator Used to Set the EAR
Accretion. Since there are no data on serum Pi or phosphorus balance and limited information on bone mineral content for children aged 1 through 3 years, a surrogate indicator had to be adopted to set the EAR. An estimation of body accretion was used based on known tissue composition and growth rates. Rates of accretion of phosphorus in bone and soft tissue (for example, lean mass) during this period of growth were then corrected for predicted efficiency of absorption and urinary losses to derive an EAR using the factorial approach.
The phosphorus requirement for bone growth can be estimated from one of two methods: (1) the phosphorus content of bony tissue gained over this age range as derived from the body composition of Fomon et al. (1982), or (2) the known increments in whole body bone mineral content using the method of dual energy x-ray absorptiometry (DXA) (Ellis et al., 1997). For both approaches, a value of 19 percent by weight was used as the phosphorus content of bone. The phosphorus content of lean tissue is assumed to be 0.23 percent based on known composition of muscle (Pennington, 1994). The computations for tissue accretion are summarized in Table 5-3. The overall estimated mean value for both sexes combined is 54 mg (1.74 mmol) phosphorus accreted per day.
The value derived for phosphorus accretion in lean and osseous tissue is supported by estimates of phosphorus retention, 10 g (323 mmol)/kg body weight gained, derived from balance studies in children aged 4 to 12 years (Fomon et al., 1982), when corrected to the average weight gain for children aged 1 through 3 years. When males and females are averaged, a total of 2.36 kg of weight is gained over this period (Fomon et al., 1982); thus a total phosphorus increment of 23.6 g (768 mmol) is gained. A daily accretion of phosphorus is then predicted to be 62 mg (2.0 mmol), which is close to that calculated above for lean plus osseous tissue accretion.
The value derived for phosphorus accretion was then employed in a factorial model to obtain the EAR. It is known that phosphorus in urine increases with phosphorus intake, described by an equation derived by Lemann (1996) in adults. This approach was adopted here since no such relationship has been developed for children. Using the equation: Purine = 1.73 + 0.512 × Pintake (in mmol/day), and an intake of 310 mg (10 mmol)/day, predicted urinary
TABLE 5-3 Accretion of Phosphorus (P) in Bone and Lean Tissue of Children Aged 1 to 3 Years Using Growth and Body Composition Data from Fomon et al. (1982)
|
Gender/Age (y) |
Change in Weight (g/y) |
Change in Lean (FFBM-osseous) (g/y) |
Lean-Tissue Pa (g/y) |
Osseous P (g/y) |
Bone Pb (g/y) |
Total P Gainc (mg/d [mmol/d]) |
|
Males |
||||||
|
1–2 |
2,440 |
1,539 |
3.54 |
94 |
17.8 |
58 (1.9) |
|
2–3 |
2,085 |
2,001 |
4.60 |
84 |
16.0 |
56 (1.8) |
|
Females |
||||||
|
1–2 |
2,730 |
2,397 |
5.51 |
75 |
14.2 |
54 (1.7) |
|
2–3 |
2,190 |
1,950 |
4.48 |
67 |
12.7 |
47 (1.5) |
|
Mean, both sexes |
54 (1.7) |
|||||
|
NOTE: Based on recent data (Ellis et al., 1997), the daily accretion of phosphorus in bone is calculated from cross-sectional measures of whole body bone mineral content in children. The values are almost identical to those calculated using Fomon et al. (1982) body composition data. Compared were the values listed vertically in the Bone P column above, the data from Ellis yield values of 16 g and 14 g for males, and 15 g and 12 g for females, at the respective ages. This comparability of values obtained by two very different methods gives credence to the method applied for this report in deriving the EARs. a Assuming a phosphorus content of soft tissue of 0.23 percent. b Assuming a phosphorus content of bone of 19 percent. c Calculated from sum of accretion of P in lean and bone/year divided by 365 days. |
||||||
excretion would be 213 mg (6.9 mmol)/day. At this intake of phosphorus, obligatory losses and predicted accretion values will be generously covered. Phosphorus intakes in excess of this amount would simply lead to increased urinary loss. When urinary excretion is added to the accrued phosphorus of 54 mg (1.74 mmol)/day (Table 5-3), there is a daily need for dietary phosphorus of 267 mg (8.6 mmol)/day.
A conservative estimate of efficiency of phosphorus absorption of 70 percent was used, as suggested for children aged 9 through 18 years, which is slightly higher than the 60 percent figure for adults (Lemann, 1996). No data are available that provide a value for percent efficiency of absorption from the typical mixed diet in early childhood. Using the equation: EAR = (accretion + urinary loss)
divided by fractional absorption, an EAR of 380 mg (12.3 mmol)/day is derived by the factorial approach.
EAR Summary: Ages 1 through 3 Years
Based on the factorial estimate, an EAR of 380 mg (12.3 mmol)/day is set for children ages 1 through 3 years.
|
EAR for Children |
1 through 3 years |
380 mg (12.3 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), the value derived for the EAR of 380 mg (12.3 mmol)/day represents a low dietary intake of phosphorus, as it falls below the first percentile (416 mg [13.4 mmol]/day) for phosphorus intake for children aged 1 through 3 years (see Appendix D). The EAR value will provide for the calculated physiological need for phosphorus accretion in lean and bone mass, accounting for the expected urinary phosphorus loss at that dietary intake. Because urinary excretion rises linearly with increasing dietary intake of phosphorus, it does not seem appropriate to set the EAR at an amount that exceeds the physiological needs for growth and maintenance.
Determination of the Recommended Dietary Allowance: Ages 1 through 3 Years
The variance in requirements cannot be determined from the available data. Thus, a coefficient of variation (CV) of 10 percent (1 standard deviation [SD]) is assumed, which results in an Recommended Dietary Allowance (RDA) of 460 mg (14.8 mmol)/day.
|
RDA for Children |
1 through 3 years |
460 mg (14.8 mmol)/day |
Ages 4 through 8 Years
Indicator Used to Set the EAR
Accretion. The rationale for using a factorial approach based on the surrogate criteria of phosphorus accretion of bone and soft tissue is the same for children ages 4 through 8 years as for children ages 1 through 3 years. The surrogate indicator of tissue accretion was used since there are no data on phosphorus balance in this age group, with the exception of a study by Wang et al. (1930). These
TABLE 5-4 Accretion of Phosphorus (P) in Bone and Lean Tissue of Children Aged 4 to 8 Years Using Growth and Body Composition Data from Fomon et al. (1982)
|
Gender/Age (y) |
Change in Weight (g/y) |
Change in Lean (FFBM-osseous) (g/y) |
Lean-Tissue Pa (g/y) |
Osseous P (g/y) |
Bone Pb (g/y) |
Total P Gainc (mg/d [mmol/d]) |
|
Males |
||||||
|
4–6 |
2,000 |
1,841 |
4.2 |
89 |
16.9 |
58 (1.9) |
|
6–8 |
2,305 |
1,957 |
4.5 |
99 |
18.8 |
64 (2.1) |
|
Females |
||||||
|
4–6 |
1,780 |
1,500 |
3.45 |
54 |
10.3 |
38 (1.2) |
|
6–8 |
2,660 |
1,647 |
3.79 |
57 |
10.8 |
40 (1.3) |
|
Mean, both sexes |
50 (1.6) |
|||||
|
NOTE: Based on recent data (Ellis et al., 1997), the daily accretion of phosphorus in bone is calculated from cross-sectional measures of whole body bone mineral content in children. The values are almost identical to those calculated using Fomon et al. (1982) body composition data. Compared were the values listed vertically in the Bone P column above, the data from Ellis yield values of 16 g and 14 g for males, and 15 g and 12 g for females, at the respective ages. This comparability of values obtained by two very different methods gives credence to the method applied for this report in deriving the EARs. a Assuming a phosphorus content of soft tissue of 0.23 percent. b Assuming a phosphorus content of bone of 19 percent. c Calculated from sum of accretion of P in lean and bone/year divided by 365 days. |
||||||
data were thought to be inadmissible since the variations in intake were accomplished by adding a calcium and phosphorus salt, and the methods used to measure phosphorus were not those used currently and may have led to analytical differences that would have made the data not applicable today. No data are available on serum phosphorus or phosphorus balance at various phosphorus intakes in children aged 4 through 8 years.
To estimate tissue accretion of phosphorus, the compositions of lean and osseous tissue were calculated based on body weight of children growing from 4 through 8 years and known content of phosphorus in these tissues. (The computations are summarized in Table 5-4.) In calculating the accretion of phosphorus over this age interval, it is apparent that there are not great differences in phosphorus accumulation between ages 4 to 6 and 6 to 8 years. This
provides support for combining the ages of 4 through 8 years in terms of phosphorus needs. The overall mean value for males of 62 mg (2.0 mmol)/day phosphorus accrued is in the same range as the value 68.2 mg (2.2 mmol)/day computed from the phosphorus composition of weight gain from Sheikh et al. as cited in the review by Lemann (1996). The slightly lower value of 40.8 mg (1.3 mmol)/day for females presumably reflects the differences in the amount of bone and lean tissue between the sexes at this age interval.
The value derived for phosphorus accretion of lean and osseous tissue is supported by estimates of phosphorus retention from balance studies in children aged 4 to 12 years; that is, phosphorus retention of 10 g (323 mmol)/kg body weight gained (Fomon et al., 1982), when applied to the average weight gain over this age interval. For males, ~2.15 kg are gained each year over this period (Fomon et al., 1982); thus a total increment of 21.6 g (697 mmol) of phosphorus is gained each year. Daily accretion of phosphorus is then predicted to be 60 mg (1.9 mmol). For females, daily phosphorus accretion calculated in this manner would be 62 mg (2.0 mmol). These estimates are similar to those calculated by summing phosphorus accretion in lean and bony compartments (Table 5-4).
For the factorial estimate of the EAR for children ages 4 through 8 years, as outlined previously for children ages 1 through 3 years, and an accretion value of 62 mg (2.0 mmol)/day, a value of 405 mg (13.1 mmol)/day was derived. The assumptions for efficiency of phosphorus absorption and urinary loss of phosphorus are identical to that used for the 1 through 3 years age group.
EAR Summary: Ages 4 through 8 Years
The estimated EAR for boys and girls ages 4 through 8 years is 405 mg (13.1 mmol)/day.
|
EAR for Children |
4 through 8 years |
405 mg (13.1 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), this value derived for the EAR again represents a low dietary intake of phosphorus, as it falls below the first percentile of intake, 537 mg (17.3 mmol)/day, for phosphorus intakes of children aged 4 through 8 years (see Appendix D). Since excess phosphorus intake will be excreted, consuming intakes in excess of physiological needs by such a magnitude is not likely of any consequence.
Determination of the RDA: Ages 4 through 8 Years
The variance in requirements cannot be determined from the available data. Thus, a CV of 10 percent (1 SD) is assumed, resulting in an RDA of 500 mg (16.1 mmol)/day.
|
RDA for Children |
4 through 8 years |
500 mg (16.1 mmol)/day |
Ages 9 through 13 and 14 through 18 Years
Indicators Used to Set the EAR
During the rapid growth period of adolescence, the most logical basis for estimating the phosphorus requirement would be from observation of the balance plateau, that is, the intake level above which no further phosphorus retention occurs, just as was done for the calcium requirement (see Chapter 4). Unfortunately, only a few phosphorus balance studies have been conducted in this age group, and insufficient data exist across a range of intakes to determine maximal retention. As an alternative, the same approach using tissue accretion used for the 1 through 3 and 4 through 8 years age groups was employed.
Accretion. Phosphorus intakes necessary to meet the needs for the addition of bone and soft tissue during this period of rapid growth can be calculated and adjusted for by urinary output and absorptive efficiency. The main limitation of this approach for this age category is that tissue accretion values are not available for adolescents beyond 14 years; thus, predicted needs for older adolescents may not be optimal to support any growth spurts beyond this age.
To estimate phosphorus requirement from tissue accretion, longitudinal data and a large database of cross-sectional data are available and represent very recent databases. Slemenda and colleagues (1994) conducted a 3-year longitudinal study in 90 white children aged 6 to 14 years at baseline. They showed increases in weight during this time of 10.9 ± 4.3 kg for the 44 prepubertal children, 19.6 ± 0.9 kg for the 38 peripubertal children, and 6.98 ± 4.54 kg for the 8 postpubertal children. The growth rate is higher and later in boys than in girls. Weight gain during this period amounts to approximately 50 percent of the ideal adult weight. However, there is enormous variability in the timing and extent of this growth acceleration.
To estimate tissue accretion of phosphorus, knowledge of both
TABLE 5-5 Accretion of Phosphorus (P) in Bone and Lean Tissue During Peak Adolescent Growth Spurt
|
Gender/Age (y) |
Change in Weighta (g/y) |
Change in Leanb (FFBM-osseous) (g/y) |
Lean-Tissue Pc (g/y) |
Osseous Pd (g/y) |
Bone Pe (g/y) |
Total P Gainf (mg/d [mmol/d]) |
|
Males |
||||||
|
13.3 |
5,000 |
3,970 |
9.1 |
320 |
60.8 |
192 (6.2) |
|
Females |
||||||
|
11.4 |
5,000 |
3,750 |
8.6 |
240 |
45.6 |
149 (4.8) |
|
Mean, both sexes |
171 (5.5) |
|||||
|
a See Table 1-3. b Body fat values from Deurenberg et al., 1990. c Assuming a phosphorus content of soft tissue of 0.23 percent. d Martin et al., 1997. e Assuming a phosphorus content of bone of 19 percent. f Calculated from sum of accretion of P in lean and bone/year divided by 365. |
||||||
lean and osseous tissue gains is required. Gains in lean mass were determined by subtracting fat from total weight gain. Percentage body fat for pubertal boys aged 13.2 (standard error [SE] 1.3) was 14.2 (95 percent confidence interval [CI] 13.0 to 15.4), and 20.2 (95 percent CI 19.3 to 21.1) for pubertal girls aged 10.5 (SE 1.6) (Deurenberg et al., 1990). Osseous tissue gains were based on a study of 228 children in Canada where mean peak bone mineral content velocity was 320 g/year in boys at age 13.3 years and 240 g/year in girls at age 11.4 years (Martin et al., 1997). The computations are summarized in Table 5-5. Assuming a phosphorus content of bone mineral of 19 percent and a phosphorus content of soft tissue of 0.23 percent (Pennington, 1994), daily phosphorus needs during peak growth would approximate 200 mg (6.5 mmol) for boys and 150 mg (4.8 mmol) for girls.
Similar to younger children, the value derived for phosphorus excretion was then employed in a factorial model to obtain the EAR. Urinary phosphate excretion rises linearly with phosphorus intake according to the equation derived by Lemann (1996) in adults. This approach was adopted here since no such relationship has been developed for adolescents. Using the equation: Purine = 1.73 + 0.512 × Pintake (in mmol/day), and an intake of 1,000 mg
(32.3 mmol)/day, predicted urinary excretion would be 565 mg (18.2 mmol)/day. When urinary phosphorus is added to the mean accrued phosphorus for both boys and girls of 175 mg (5.6 mmol) (Table 5-5), there is a daily need for dietary phosphorus of 740 mg (23.9 mmol)/day. Absorption efficiency in the few balance studies performed in this age group (Greger et al., 1978; Lutwak et al., 1964) averaged 60 to 80 percent. This is consistent with the range of absorption efficiencies (60 to 65 percent) found in adults (Lemann, 1996). Using a midpoint value of absorption efficiency of 70 percent, ingested phosphorus to cover tissue accretion and urinary loss would need to be 1,055 mg (34 mmol)/day for both boys and girls. Although these phosphorus intakes cover tissue accumulation needs of the observed average adolescent, these intakes may not be optimal at the peak of the adolescent growth spurt.
The small amount of balance data available supports this estimate of phosphorus needs for children up to 14.5 years of age. Greger et al. (1978) studied 14 girls aged 12.5 to 14.5 years. Their balances averaged +82 ± 124 mg (+2.6 ± 4 mmol)/day on phosphorus intakes of 820 ± 10 mg (26.5 ± 0.3 mmol)/day. On phosphorus intakes of 925 mg (29.8 mmol)/day, 8 of 11 girls aged 12.5 to 14.2 years were in positive phosphorus balance with zinc intakes of 11.3 mg/day (Greger et al., 1979). In other studies, five adolescents aged 9 to 13 years and 18 adolescents aged 8 to 11 years were in positive phosphorus balance at intakes exceeding 1 g (32.3 mmol)/day (Lutwak et al., 1964; Sherman and Hawley, 1922). Of these 23 individuals, 4 boys and 13 girls aged 8 to 12 years were in positive phosphorus balance at daily phosphorus intakes above 34.5 mg (1.1 mmol)/kg body weight or 693 mg (22.4 mmol).
Serum Pi. An attempt was also made to estimate the EAR during adolescence by using the approach employed for adults, for example, using ECF Pi as the functional indicator, and estimating the intake needed to sustain ECF Pi at the lower limit of normal for the age concerned (see below for corresponding approach in adults). As Table 5-1 shows, the interpolated 2.5 percentile for Pi at age 9 is approximately 1.25 mmol/liter (3.9 mg/dl), falling to approximately 1.05 mmol/liter (3.3 mg/dl) by age 18. The curve in Figure 5-1 relates to adults and hence cannot be used directly. However, the lack of effective regulation of ECF Pi and the fact that it rises and falls with intake means that the rise in Pi for any given increment will be a function of the volume of the ECF into which the absorbed phosphorus is inserted, or in other words, a function of body size. Thus, a straightforward adjustment for body weight may permit a
first approximation of the requirement for this age group. A number of assumptions related to serum Pi during adolescence and growth needs were necessary to complete this calculation, and the resulting value was not dissimilar from that determined using tissue accretion and the factorial method.
EAR Summary: Ages 9 through 13 and 14 through 18 Years
The EAR for children ages 9 through 13 years can be set based on the factorial approach at 1,055 mg (34 mmol)/day. Although accretion data are not available for ages 14 through 18 years, it seems reasonable to maintain this EAR value for the older adolescent since a similar value was obtained using the serum phosphorus curve extrapolated from adults. It should be noted, however, that this age range (9 through 18 years) brackets a period of intense growth, with growth rate, absorption efficiency, and normal values for ECF Pi changing during this time. Because of the similarity of these sexspecific estimates and the uncertainties involved, a single value of 1,055 mg (34 mmol)/day is selected for both males and females ages 9 through 18 years.
|
EAR for Boys |
9 through 13 years |
1,055 mg (34 mmol)/day |
|
14 through 18 years |
1,055 mg (34 mmol)/day |
|
|
EAR for Girls |
9 through 13 years |
1,055 mg (34 mmol)/day |
|
14 through 18 years |
1,055 mg (34 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), this EAR of 1,055 mg (34 mmol)/day is slightly below the twenty-fifth percentile (1,090 mg [35.2 mmol]) of phosphorus intake for boys, aged 9 through 13 years, and slightly above the twenty-fifth percentile of intake (1,005 mg [32.4 mmol]) for girls in this same age range (see Appendix D). For boys ages 14 through 18, the EAR of 1,055 mg (34 mmol)/day is close to the tenth percentile (1,072 mg [34.6 mmol] ) of phosphorus intake. The EAR of 1,054 mg (34 mmol)/day for girls ages 14 through 18 is slightly below the fiftieth percentile (1,097 mg [35.4 mmol]) of phosphorus intake.
Determination of the RDA: Ages 9 through 13 and 14 through 18 Years
The variance in requirements cannot be determined from the available data. Thus, a CV of 10 percent (1 SD) is assumed. This results in a RDA for phosphorus of 1,250 mg (40.3 mmol)/day for girls and boys ages 9 through 18 years.
TABLE 5-6 Phosphorus Intakes Related to Specific Serum Inorganic Phosphorus Values in Adults
|
Serum Pi (mmol/liter) |
Absorbed Intake (mmol/day) |
Ingested Intakea (mmol/day) |
|
0.87 |
11.6 |
18.6 |
|
1.00 |
23.9 |
38.2 |
|
1.15 |
43.2 |
69.2 |
|
1.40 |
71.0 |
113.6 |
|
a Ingested intake given as (absorbed intake)/0.625. |
||
|
RDA for Boys |
9 through 13 years |
1,250 mg (40.3 mmol)/day |
|
14 through 18 years |
1,250 mg (40.3 mmol)/day |
|
|
RDA for Girls |
9 through 13 years |
1,250 mg (40.3 mmol)/day |
|
14 through 18 years |
1,250 mg (40.3 mmol)/day |
Ages 19 through 30 and 31 through 50 Years
Indicator Used to Set the EAR
Serum Pi. The relationship between serum Pi and absorbed intake, as presented in Figure 5-1, allows estimation of the intakes associated with Pi values within the range typically considered normal.
The extrapolation from absorbed intake to ingested intake shown in Table 5-6 is based on an absorption efficiency for phosphorus of 60 to 65 percent, the value typically observed in studies of adults on mixed diets (Heaney and Recker, 1982; Stanbury, 1971; Wilkinson, 1976). This absorption estimate for phosphorus is fairly robust, since variation in absorptive performance for phosphorus in adults is narrow (Heaney and Recker, 1982; Wilkinson, 1976).
The estimates of ingested intake in Table 5-6 apply to a typical, mixed diet. They will underestimate intakes needed to achieve a given serum Pi value if the dietary phosphorus consists heavily of phytate phosphorus. Thus, diets in which major fractions of the phosphorus intake are derived from unleavened cereal sources will require higher intakes to produce the curve of Figure 5-1 (which, as already noted, is for typical mixed diets), that is, the curve will be expanded to the right when the x-axis is expressed as ingested intake. This is shown by the lower dashed curve in Figure 5-1. Conversely, diets in which much of the phosphorus is derived, for example, from the phosphoric acid in certain car-
bonated beverages, and in which there is correspondingly little coingested calcium, may be expected to yield the curve of Figure 5-1 at lower phosphorus intakes; that is, the curve will shrink to the left—the upper dashed curve.
EAR Summary: Ages 19 through 30 and 31 through 50 Years
These latter considerations aside, using the lower end of the normal adult Pi range (0.87 mmol/liter [2.7 mg/dl]) yields an ingested intake value of ~580 mg (~19 mmol)/day, which may be the best available EAR for adults. By definition, roughly half of all adults would not be able to maintain a Pi of 0.87 mmol/liter (2.7 mg/dl) at this intake. (Importantly, while most adults have fasting serum Pi values above 1.0 mmol/liter [3.1 mg/dl], that fact cannot be used to establish the requirement, since intake in excess of the requirement will elevate the serum Pi.) If, alternatively, the middle of the normal range for serum Pi (1.15 mmol/liter [3.6 mg/dl]) is taken as the basis for the EAR, a much higher value, ~2,100 mg (~68 mmol)/day results. Since the slope of the curve of Figure 5-1 is very gradual above the renal threshold, intake estimates will be very sensitive to the value of serum Pi selected. Thus, the EAR for both men and women ages 19 through 50 is set at 580 mg (18.7 mmol)/day.
|
EAR for Men |
19 through 30 years |
580 mg (18.7 mmol)/day |
|
31 through 50 years |
580 mg (18.7 mmol)/day |
|
|
EAR for Women |
19 through 30 years |
580 mg (18.7 mmol)/day |
|
31 through 50 years |
580 mg (18.7 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), all males aged 19 through 50 years have phosphorus intakes above the EAR of 580 mg (18.7 mmol)/day (see Appendix D). The median intake for men, aged 19 through 30 years, is 1,613 mg (52.0 mmol)/day, and the first percentile of intake is 809 mg (26.1 mmol)/day. For men, aged 31 through 50 years, the median intake of phosphorus is 1,484 mg (47.9 mmol)/day, and the first percentile of intake is 705 mg (22.7 mmol)/day.
For women aged 19 through 30 years, the median phosphorus intake is 1,005 mg (32.4 mmol)/day, and the EAR of 580 mg (18.7 mmol)/day is at the first percentile of intake, 580 mg (18.7 mmol)/day. For women aged 31 through 50 years, the median phosphorus intake is 990 mg (31.9 mmol)/day. The EAR in this group of wom-
en is slightly below fifth percentile of intake, 593 mg (19.1 mmol)/day, based on the 1994 CSFII data.
Determination of the RDA: Ages 19 through 30 and 31 through 50 Years
The variance in requirements cannot be determined from the available data. Thus, a CV of 10 percent (1 SD) is assumed, resulting in an RDA of 700 mg (22.6 mmol)/day.
|
RDA for Men |
19 through 30 years |
700 mg (22.6 mmol)/day |
|
31 through 50 years |
700 mg (22.6 mmol)/day |
|
|
RDA for Women |
19 through 30 years |
700 mg (22.6 mmol)/day |
|
31 through 50 years |
700 mg (22.6 mmol)/day |
Ages 51 through 70 and > 70 Years
The data on which the foregoing phosphorus analyses were based were derived from adults of mixed ages, including 51 through 70 and > 70 years. Data specific to these ages are not available. Intestinal absorption efficiency for phosphorus is not known to change appreciably with age, and as noted above, changes in the renal clearance of phosphorus are not sufficient to alter the curve of Figure 5-1 until GFR is reduced by approximately 80 percent. Hence, it is reasonable to adopt the same phosphorus EAR for older adults as for younger adults.
EAR Summary: Ages 51 through 70 and > 70 Years
The EAR for both men and women ages 51 years and older is set at 580 mg (18.7 mmol)/day.
|
EAR for Men |
51 through 70 years |
580 mg (18.7 mmol)/day |
|
> 70 years |
580 mg (18.7 mmol)/day |
|
|
EAR for Women |
51 through 70 years |
580 mg (18.7 mmol)/day |
|
> 70 years |
580 mg (18.7 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), the median phosphorus intake for men, aged 51 through 70 years, is 1,274 mg (41.1 mmol)/day (see Appendix D). All of the men, aged 51 through 70 years, had phosphorus intakes in amounts above the EAR. The median intake for men,
aged 71 years and older, is 1,176 mg (37.9 mmol)/day and the first percentile of intake is 559 mg (18.0 mmol)/day, which is close to their EAR of 580 mg (18.7 mmol)/day.
The median phosphorus intake for women aged 51 through 70 years is 966 mg (31.2 mmol)/day, based on the adjusted 1994 CSFII intake data. The EAR of 580 mg (18.7 mmol)/day for these women would fall close to the fifth percentile of intake, 599 mg (19.3 mmol)/day. The median phosphorus intake for women, aged 71 years and older, is 859 mg (27.7 mmol)/day, and the tenth percentile of phosphorus intake is 588 mg (19.0 mmol)/day, which is slightly above the EAR of 580 mg (18.7 mmol)/day.
Determination of the RDA: Ages 51 through 70 and >70 Years
The variance in requirements cannot be determined from the available data. Thus a CV of 10 percent (1 SD) is assumed, resulting in an RDA for phosphorus of 700 mg (22.6 mmol)/day for men and women ages 51 years and older.
|
RDA for Men |
51 through 70 years |
700 mg (22.6 mmol)/day |
|
> 70 years |
700 mg (22.6 mmol)/day |
|
|
RDA for Women |
51 through 70 years |
700 mg (22.6 mmol)/day |
|
> 70 years |
700 mg (22.6 mmol)/day |
Pregnancy
Indicator Used to Set the EAR
Phosphorus Content. Phosphorus content of the term infant at birth is 17.1 g (552 mmol), with 88.3 percent of phosphorus accounted for in bone and water (Fomon et al., 1982). The major physiological adaptations of the mother to meet the increased need for calcium to support fetal growth also should supply the fetus with a sufficient amount of phosphorus. The increased efficiency in intestinal absorption of calcium resulting from increased 1,25(OH)2D concentrations also will lead to increased intestinal absorption of phosphorus.
Balance studies in 24 pregnant women demonstrated positive phosphorus balance, which increased with length of pregnancy (Heaney and Skillman, 1971). Net phosphorus absorption in these women averaged 70 percent, compared with absorption in the range of 60 to 65 percent typically found in nonpregnant adults (Heaney
and Recker, 1982; Stanbury, 1971; Wilkinson, 1976). Daily fetal phosphorus requirements of about 62 mg (2 mmol) are needed to produce a term infant at birth with a phosphorus content of 17.1 g (552 mmol) (Fomon et al., 1982). The EAR for phosphorus in adults ages 19 through 30 years is approximately 580 mg (18.7 mmol)/day at an absorption of 60 percent (resulting in an absorbed phosphorus of 353 mg [11.4 mmol/day]). Assuming a 70 percent absorption of phosphorus during pregnancy (Heaney and Skillman, 1971), a similar EAR of 580 mg (18.7 mmol)/day would lead to an absorbed phosphorus of 412 mg (13.3 mmol)/day. This increase in absorbed phosphorus during pregnancy (59 mg [1.9 mmol/day]) approximately equals the estimated fetal phosphorus requirement of 62 mg (2 mmol)/day.
Serum phosphorus concentrations during pregnancy are within the normal range at mean daily intakes of approximately 1,550 mg (50 mmol) (95 percent CI range of 1,260 to 1,840 mg [40.6 to 59.4 mmol]) (Cross et al., 1995a). No studies have specifically investigated the effect of dietary intake of phosphorus on phosphorus balance during pregnancy.
EAR and RDA Summary for Pregnancy
No evidence at this time supports an increase of the EAR and RDA during pregnancy above the level recommended during the nonpregnant state. Intestinal absorption of phosphorus increases by about 10 percent during pregnancy (Heaney and Skillman, 1971). That change should be sufficient to provide the necessary phosphorus for fetal growth.
|
EAR for Pregnancy |
|
|
14 through 18 years |
1,055 mg (34.0 mmol)/day |
|
19 through 30 years |
580 mg (18.7 mmol)/day |
|
31 through 50 years |
580 mg (18.7 mmol)/day |
|
RDA for Pregnancy |
|
|
14 through 18 years |
1,250 mg (40.3 mmol)/day |
|
19 through 30 years |
700 mg (22.6 mmol)/day |
|
31 through 50 years |
700 mg (22.6 mmol)/day |
Utilizing the 1994 CSFII intake data for 33 pregnant women, adjusted for day-to-day variations (Nusser et al., 1996), the value derived for the EAR of 580 mg (18.7 mmol)/day for pregnant women ages 19 through 50 years represents a low dietary intake of phos-
phorus, as it falls below the first percentile of intake of 773 mg (24.9 mmol)/day (see ). The EAR of 1,055 mg (34 mmol) for pregnant women ages 14 through 18 years falls slightly above the fifth percentile of intake of 1,012 mg (32.6 mmol)/day.
Special Considerations
Adolescent Mothers and/or Multiple Fetuses. It is not known whether phosphorus requirements are increased in the adolescent mother and in mothers pregnant with more than one fetus. These conditions lead to increased maternal or fetal needs for phosphorus that may not be met by increased intestinal absorption. The mediating role of maintaining calcium homeostasis is also important in these situations: a diet insufficient in calcium may lead to increased PTH concentrations and decreased renal tubular reabsorption of phosphorus.
Lactation
Indicators Used to Set the EAR
Human Milk. Concentrations of phosphorus in human milk range from approximately 3.9 to 5.1 mmol/liter (12.1 to 15.8 mg/dl) and decrease as lactation progresses (Table 5-2). Assuming a milk production of 780 ml/day, a lactating woman may lose approximately 90 to 120 mg (2.9 to 3.9 mmol)/day of phosphorus in her milk. No studies have investigated the effect of varying phosphorus intake on phosphorus homeostasis during lactation.
Serum Pi. Despite the loss of phosphorus in milk, serum phosphorus concentrations in lactating women are in the high-normal or above-normal range, and they are higher in lactating women than in nonlactating women (Figure 5-2) (Byrne et al., 1987; Chan et al., 1982a; Cross et al, 1995a; Dobnig et al., 1995; Kalkwarf et al., 1996; Kent et al., 1990; Lopez et al., 1996; Specker et al., 1991a). This high serum phosphorus occurs at a time when there is an increase in bone resorption that appears to be related to non-dietary factors (see Chapter 4). The elevated serum phosphorus is due in whole or part to the fall in serum PTH which leads to high serum Pi.
EAR and RDA Summary for Lactation
Currently no evidence supports that phosphorus requirements are
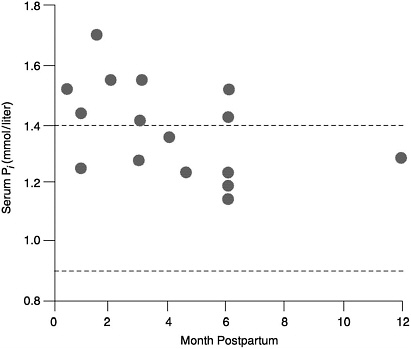
FIGURE 5-2 Serum phosphorus concentrations in lactating and non-lactating women. The dotted lines represent the normal range for non-lactating women. The circles represent the serum phosphorus levels of lactating women (Bryne et al., 1987; Chan et al., 1982b; Cross et al., 1995a; Dobnig et al., 1995; Kalkwarf et al., 1996; Kent et al., 1990; Lopez et al., 1996; Specker et al., 1991a).
increased during lactation. Apparently, increased bone resorption and decreased urinary excretion of phosphorus (Kent et al., 1990), which occur independent of dietary intake of phosphorus or calcium, provide the necessary phosphorus for milk production. Therefore, the EAR and RDA are estimated to be similar to that obtained for nonlactating women of their respective age groups.
|
EAR for Lactation |
|
|
14 through 18 years |
1,055 mg (34.0 mmol)/day |
|
19 through 30 years |
580 mg (18.7 mmol)/day |
|
31 through 50 years |
580 mg (18.7 mmol)/day |
|
RDA for Lactation |
|
|
14 through 18 years |
1,250 mg (40.3 mmol)/day |
|
19 through 30 years |
700 mg (22.6 mmol)/day |
|
31 through 50 years |
700 mg (22.6 mmol)/day |
Utilizing the 1994 CSFII intake data, adjusted for day-to-day variations (Nusser et al., 1996), the median intake of phosphorus for the 16 lactating women in the survey is 1,483 mg (47.8 mmol)/day and the lowest intake reported was at the first percentile of 1,113 mg (35.9 mmol)/day (see Appendix D). All women consumed above the phosphorus EAR of 580 to 1,055 mg (18.7 to 34 mmol)/day during lactation.
Special Considerations
Adolescent Mothers and Mothers Nursing Multiple Infants. As with calcium, phosphorus requirements may be increased in lactating adolescents and in mothers nursing multiple infants. Requirements during these periods may be higher because of the adolescent mother 's own growth requirements and the requirement for increased milk production while nursing multiple infants. It is not known whether decreased urinary phosphorus and increased maternal bone resorption provides sufficient amounts of phosphorus to meet these increased needs.
TOLERABLE UPPER INTAKE LEVELS
Hazard Identification
As shown in Figure 5-1, serum Pi rises as total phosphorus intake increases. Excess phosphorus intake from any source is expressed as hyperphosphatemia, and essentially all the adverse effects of phosphorus excess are due to the elevated Pi in the ECF. The principal effects that have been attributed to hyperphosphatemia are: (1) adjustments in the hormonal control system regulating the calcium economy, (2) ectopic (metastatic) calcification, particularly of the kidney, (3) in some animal models, increased porosity of the skeleton, and (4) a suggestion that high phosphorus intakes could reduce calcium absorption by complexing calcium in the chyme. Concern about high phosphorus intake has been raised in recent years because of a probable population-level increase in phosphorus intake through such sources as cola beverages and food phosphate additives.
It has been reported that high intakes of polyphosphates, such as are found in food additives, can interfere with absorption of iron, copper, and zinc (Bour et al., 1984); however, described effects are small, and have not been consistent across studies (for example,
Snedeker et al., 1982). For this reason, as well as because trace mineral status may be low for many reasons, it was not considered feasible to use trace mineral status as an indicator of excess phosphorus intake. Nevertheless, given the trend toward increased use of phosphate additives in a variety of food products (Calvo and Heath, 1988; Calvo and Park, 1996), it would be well to be alert to the possibility of some interference in individuals with marginal trace mineral status.
Most of the studies that describe harmful effects of phosphorus intake used animal models. In extrapolating these data to humans, it is important to note that the phosphorus density of human diets represents the extreme low end of the continuum of standard diets for pets and laboratory animals. The median human dietary phosphorus density in the 1994 CSFII was very close to 62 mg (2.0 mmol)/100 kcal for all adults and for both sexes (Cleveland et al., 1996). By contrast, the diets of standard laboratory rats and mice have phosphorus densities ranging from 124 to 186 mg (4 to 6 mmol)/100 kcal. Cats and dogs have diets with densities close to 279 mg (9 mmol)/100 kcal, and laboratory primate diets average about 155 mg (5 mmol)/100 kcal.
Adjustments in Calcium-Regulating Hormones
As noted earlier, Pi is not, strictly speaking, regulated. Thus, a high phosphorus diet produces a higher level of plasma Pi, especially during the absorptive phase after eating. High Pi levels reduce urine calcium loss, reduce renal synthesis of 1,25(OH) 2D, reduce serum ionized calcium, and lead to increases in PTH release (Portale et al., 1989; Wood et al., 1988). These effects reflect adjustments in the control system that regulates the calcium economy and are not in themselves necessarily adverse. As already noted, the reduction in 1,25(OH)2D synthesis may slightly mitigate the hyperphosphatemia by a small reduction in phosphorus absorption from the intestine.
It is known that added dietary phosphate loads of 1.5 to 2.5 g of inorganic phosphorus (as phosphate) in humans lead acutely to very slight drops in ECF [Ca2+] and to correspondingly elevated PTH concentrations (Calvo and Heath, 1988; Silverberg et al., 1986). It has been proposed that these adjustments in circulating calcium regulating hormones (associated with any elevation of serum Pi, even though within the usual normal range) could have adverse effects on the skeleton (Calvo and Heath, 1988; Calvo et al., 1988, 1990; Krook et al., 1975). The increase in PTH is often pre-
sumed to be harmful to bone. The mild hypocalcemic effect seen with acute increases in phosphorus intake is often attributed to the formation of calcium phosphate (CaHPO4) complexes in plasma, which is the presumed mechanism driving down [Ca2+] (Krook et al., 1975). However, it is doubtful that this is the correct explanation. As already noted, adult plasma is undersaturated with respect to CaHPO4 H2O, and the modest elevations in plasma Pi produced by a high phosphorus diet are probably not sufficient to alter plasma [Ca2+] directly. Moreover, causes of hypercalcemia, such as primary bone wasting and simple intravenous infusion of calcium, cause serum P i to rise, not fall, as might be expected if concentrations of the two ions were reciprocally related to one another at normal concentrations (Howard et al., 1953). Rather, it is more likely that the initial fall in [Ca2+] following elevation of plasma Pi is produced by direct inhibition of PTH-mediated osteoclastic release of calcium from bone (Raisz and Niemann, 1969). Thus, rather than harmful, this inhibition of bone resorption and consequent increase in PTH may actually be beneficial to bone, since it amounts to a relative resistance to the bone-resorbing effects of PTH. This point had been made by an earlier expert panel (Chinn, 1981) and is supported by more recent evidence showing that, despite the elevated PTH, urine hydroxyproline (a measure of bone resorption) falls on high phosphorus intakes, as does urine calcium (Silverberg et al., 1986).
Diets high in phosphorus and low in calcium produce a sustained rise in PTH (Calvo et al., 1988, 1990); but diets low in calcium without extra phosphorus produce the same change (Barger-Lux et al., 1995), and for that reason it is unlikely that the high phosphorus feature of the altered intake is the culprit in the first instance. In addition, with respect to high phosphorus intakes, chronic administration of 2 g (65 mmol)/day phosphorus in men for at least 8 weeks produced no effect on calcium balance or calcium absorption relative to a diet containing only 806 mg (26 mmol) phosphorus (Spencer et al., 1965, 1978a). Calcium intake (low, normal, or high) had no influence on this lack of effect, underscoring the lack of physiological relevance of the dietary Ca:P ratio in adults. Further, calcium kinetic studies performed in adult women in whom phosphorus intake was doubled from 1.1 to 2.3 g (35.5 to 74.2 mmol), showed no effect whatsoever on bone turnover processes after 4 months of treatment (Heaney and Recker, 1987). A similar conclusion was reached more recently by Bizik et al. (1996), who doubled phosphorus intake (from 800 to 1,600 mg [26 to 52 mmol]/day) for 10 days in seven healthy young men (aged 22 to 31
years) and found no increase in urine deoxypyridinoline excretion (a marker of bone resorption). For all these reasons, it is doubtful whether phosphorus intakes, within the range currently thought to be experienced by the U.S. population and/or associated with serum Pi values in the normal range, adversely affect bone health.
Normal, healthy, term-born infants, like adults, can adjust to a relatively wide range of dietary Ca:P ratios as provided in contemporary infant formulas. However, as a result of developmental immaturity in renal handling of phosphorus by infants, ECF [Ca2+] and Pi concentrations are closer to saturation in infancy, and infants are, therefore, more at risk of developing hypocalcemia as a consequence of hyperphosphatemia (DeVizia and Mansi, 1992). However, in the first month of life, some infants exhibit unusual sensitivity to phosphorus intakes above those associated with human milk. In the past, the clinical syndrome of late neonatal hypocalcemic tetany was observed when infants were fed whole evaporated cow milk with a very high phosphorus content (DeVizia and Mansi, 1992). Surprisingly, even with the introduction of modified cow milk formulas with phosphorus content reduced to one-half or less than that of evaporated whole milk, the syndrome of hypocalcemia has still been observed in young infants (Specker et al., 1991b; Venkataraman et al., 1985). However, the phosphorus content of such formulas is still substantially higher than that of human milk (Specker et al., 1991b). If such hyperphosphatemia is allowed to persist during early infancy, parathyroid hyperplasia, ectopic calcifications, and low serum calcitriol may occur (Portale et al., 1986). In such cases, the compensatory mechanisms for handling phosphate loads, mainly renal excretion, must be overwhelmed, leading to excessive phosphorus retention and the other metabolic consequences.
It has also been suggested that a high content of phosphorus and calcium in an infant's diet may be a predisposing factor for the development of retention acidosis (Manz, 1992). It is not possible to identify, in advance, infants at risk for these syndromes unless they have renal dysfunction. Based on the data of Specker et al. (1991b), the risk of hypocalcemia (serum calcium less than 1.1 mmol/liter [4.4 mg/dl]) is 30 out of 10,000 neonates fed such formulas. Human milk with its low phosphorus content is both safer and better suited to the growth needs of the infant than cow milk (Manz, 1992). Finally, one report associates high intakes of phosphoric acid-containing cola beverages with slight reductions of serum calcium in Mexican children (Mazariegos-Ramos et al., 1995), but it is not clear to what extent the effect is due to the acid load of
the colas, the associated low intake of calcium-rich beverages, or the phosphorus itself.
Except as noted in very young infants, none of these adjustments in calcium-regulating hormones is clearly adverse in its own right, particularly if calcium intake is adequate. Hence these effects do not provide a useful basis for estimating the Tolerable Upper Intake Level (UL).
Metastatic Calcification
The most serious, clearly harmful effect of hyperphosphatemia is calcification of nonskeletal tissues. This occurs when the calcium and phosphorus concentrations of ECF exceed the limits of solubility for secondary calcium phosphate (CaHPO4). This critical concentration is strongly dependent on amounts of other ions in the ECF, especially HCO3− citrate, H+, and K+, and so cannot be unambiguously defined. However, tissue calcification virtually never occurs at ECF calcium × phosphorus ion products less than ~4 (mmol/liter)2 [~1 (mg/dl)2]. Although ECF in adults is normally less than half-saturated with respect to CaHPO4, elevation of plasma Pi, if extreme, can bring the ECF to the point of saturation. Although both calcium and phosphate are involved in such ectopic mineralization, ECF calcium levels are tightly regulated and are usually affected little by even large variations in calcium intake. By contrast, the sensitivity of ECF Pi to joint effects of diet and renal clearance means that an elevation in ECF Pi will usually be the cause of supersaturation. When calcification involves the kidney, renal function can deteriorate rapidly, renal phosphorus clearance drops, and ECF Pi rises yet further, leading to a rapid downhill spiral.
Under saturated conditions, susceptible tissue matrices will begin to accumulate CaHPO4 crystals, particularly if local pH rises above 7.4. Saturation of ECF with respect to calcium and phosphorus almost never occurs in individuals with normal renal function, mainly because urine phosphate excretion rises in direct proportion to dietary intake. As Figure 5-1 shows, the upper limit of the normal adult range for serum Pi typically occurs at absorbed intakes above 2.2 g (71 mmol)/day. At 62.5 percent absorption, that means ingested intakes above 3.4 g (110 mmol)/day. The 1994 CSFII data indicate that the reported intake at the ninety-fifth percentile was 2.5 g (81.7 mmol)/day in boys aged 14 through 18 years (see Appendix D). Hyperphosphatemia from dietary causes becomes a problem mainly in patients with end-stage renal disease or in such conditions as vitamin D intoxication. When functioning kidney tissue mass is reduced to less than ~20 percent of normal, the GFR becomes too low to clear typical absorbed loads of dietary phospho-
rus, and then even sharply reduced phosphorus diets may still be excessive as they lead to hyperphosphatemia.
Although metastatic calcification can occur in patients with endstage renal disease in whom ECF Pi levels are not adequately controlled, it is not known to occur from dietary sources alone in persons with adequate renal function. For that reason, calcification in previously normal kidneys produced by high phosphorus intakes has been studied mainly in rats and mice (Craig, 1959; Hamuro et al., 1970; McFarlane, 1941; NRC, 1995). Production of calcification has required very high phosphate loads over and above the animals' already high basal phosphate intakes and in several reports has required partial reduction of renal tissue mass, as well.
Skeletal Porosity
Skeletal lesions associated with high phosphorus intakes have been described in rabbits (Jowsey and Balasubramaniam, 1972) and bulls (Krook et al., 1975). As with kidney toxicity, the bony lesions required extremely large phosphate intakes (in rabbits, about 40-fold typical human intakes on a body weight basis, and in bulls, feeding of a ration designed to support milk production in cows). None of these situations has any evident direct relevance to human nutrition or to human dietary intake of phosphate. Krook et al. (1975) also noted that bone loss develops in household pets and zoo animals fed human table scraps and meat. Despite acknowledging that such foods are poor in calcium, they attribute the bone loss to the high phosphorus content of such diets. Lacking evidence that phosphorus would produce this effect with diets adequate in calcium, this conclusion seems unwarranted. Finally, given the evidence cited above that high phosphorus intakes in humans do not lead to negative calcium balance or to increased bone resorption, it seems likely that the bone disease in other animals is more a consequence of low effective calcium intake than of high phosphorus intake per se.
Interference with Calcium Absorption
As noted, some concerns have been expressed that a high phosphorus intake could interfere with calcium nutrition by complexing calcium in the chyme and reducing its absorption (Calvo and Heath, 1988; Calvo and Park, 1996). Given the relative absorption efficiencies of calcium and phosphorus, there would not be a stoichiometric excess of phosphorus relative to calcium in the chyme until the Ca:P intake ratio fell below 0.375:1. However, even this is a purely theoretical concern. In the studies of Spencer et al. (1978a), in
which inorganic neutral phosphate was added to the diet, and of Heaney and Recker (1982), who studied women on their habitual intakes of food phosphorus, even Ca:P ratios as low as 0.08:1 did not lower calcium absorption. Nevertheless, it must be noted that it is more difficult for the body to compensate for impaired calcium absorption at low dietary calcium intakes compared with higher intakes (Heaney, 1997). As prior expert panels have noted (Chinn, 1981), even the theoretical potential for interference with the calcium economy by high phosphorus intakes is effectively negated if calcium intake is adequate.
Dose-Response Assessment
Adults: Ages 19 through 70 Years
Data Selection. A UL can be defined as an intake associated with the upper boundary of adult normal values of serum Pi. No reports exist of untoward effects following high dietary phosphorus intakes in humans. Essentially all instances of dysfunction (and, hence, all instances of hyperphosphatemia) in humans occur for nondietary reasons (for example, end-stage renal disease, vitamin D intoxication). Therefore, data on the normal adult range for serum Pi are used as the basis for deriving a UL for adults.
Identification of a No-Observed-Adverse-Effect Level (NOAEL) (or Lowest-Observed-Adverse-Effect Level [LOAEL]) and Critical Endpoint. If the normal adult range for serum Pi is used as a first approach to estimating the UL, the upper boundary of adult normal values of serum Pi is reached at a daily phosphorus intake of 3.5 g (113 mmol) (Figure 5-1). There is no evidence that individuals consuming this intake may experience any untoward effects. As shown in Table 5-1, infants, children, and adolescents have higher upper limits for serum Pi than do adults, which indicates that their tissues tolerate the higher Pi levels well. Values of Pi above the nominal adult human normal range are also normally found in typical adult laboratory animals (for example, rats) and occur regularly in adult humans treated with the bisphosphonate, etidronate, used for the treatment of Paget's disease of the bone and osteoporosis (Recker et al., 1973). No suggestion of harm comes from any of these situations, indicating that the UL is substantially higher than that associated with the upper normal bound of serum Pi in adults.
The higher values for serum Pi in infancy are manifestly tissue-safe levels, and if they are taken as an approximation of the upper normal
human value (on the ground that there is no basis for assuming major differences in tissue susceptibility to metastatic mineralization at different ages), the corresponding ingested intake in an adult (assuming the relationship of Figure 5-1) would be over 10.2 g (330 mmol)/day.
Uncertainty Assessment. No benefit is evident from serum Pi values above the usual normal range in adults. Moreover, information is lacking concerning adverse effects in the zone between normal Pi and levels associated with ectopic mineralization. Therefore, in keeping with the pharmacokinetic practice where the relationship between intake and blood level is known (Petley et al., 1995), an uncertainty factor (UF) of 2.5 is chosen.
Derivation of the UL. A UL of ~4.0 g (~130 mmol)/day for adults is calculated by dividing a NOAEL of 10.2 g (330 mmol)/day by a UF of 2.5.
|
UL for Adults |
19 through 70 years |
4.0 g (130 mmol)/day |
Infants: Ages 0 through 12 Months
As with adults, there are essentially no reports of adverse effects clearly attributable to high phosphorus intake of dietary origin in infants, children, or adolescents. Except for the sensitivity of very young infants noted above, there are no data relating to adverse effects of phosphorus intake for most of the first year of life. For that reason, it was determined that it was impossible to establish a specific UL for infants.
|
UL for Infants |
0 through 12 months |
Not possible to establish; source of intake should be from formula and food only |
Toddlers and Children: Ages 1 through 8 Years
For toddlers and children, a UL of 3.0 g (96.8 mmol)/day is calculated by dividing the NOAEL for adults (10.2 g [330 mmol]/day by a UF of ~3.3 to account for potentially increased susceptibility due to smaller body size.
|
UL for Children |
1 through 8 years |
3.0 g (96.8 mmol)/day |
Adolescents: Ages 9 through 18 Years
There is no evidence to suggest increased susceptibility to adverse effects during adolescence. Therefore, the same UL specified above for adults is selected for adolescents, 4.0 g (130 mmol)/day.
|
UL for Adolescents |
9 through 18 years |
4.0 g (130.0 mmol)/day |
Older Adults: Ages > 70
Because of an increasing prevalence of impaired renal function after age 70, a larger UF of 3.3 seems prudent, and the UL for adults of this age is set at 3.0 g (96.8 mmol)/day.
|
UL for Older Adults |
> 70 years |
3.0 g (96.8 mmol)/day |
Pregnancy and Lactation
During pregnancy, absorption efficiency for phosphorus rises by about 15 percent, and thus, the UL associated with the upper end of the normal range will be about 15 percent lower, that is, about 3.5 g (112.9 mmol)/day. During lactation, the phosphorus economy of a woman does not differ detectably from the nonlactating state. Hence the UL for this physiologic state is not different from the nonlactating state, 4.0 g (130 mmol)/day.
|
UL for Pregnancy |
14 through 50 years |
3.5 g (112.9 mmol)/day |
|
UL for Lactation |
14 through 50 years |
4.0 g (130.0 mmol)/day |
Special Considerations
It is recognized that population groups such as professional athletes, military trainees, or those whose level of energy expenditure exceeds 6,000 kcal/day, may have dietary phosphorus intakes whose distributions overlap these limits. In such individuals with phosphorus intakes above the UL, no harm is known to result.
Exposure Assessment
Utilizing the 1994 CSFII data, adjusted for one day food records (Nusser et al., 1996), the highest mean intake of phosphorus for any age and life stage group was reported for males aged 19 through 30 years: 1.7 g (53.5 mmol)/day. The highest reported intake at the
ninety-fifth percentile was 2.5 g (81.7 mmol)/day in boys aged 14 through 18 years (see Appendix D), which is well below the UL of 4.0 g (130 mmol)/day. In 1986, approximately 10 percent of adults in the United States took a supplement containing phosphorus (Moss et al., 1989), and of those, the ninetieth percentile of supplemental phosphorus intake was 264 mg (8.5 mmol)/day. The ninety-fifth percentile intake for phosphorus supplements for adults was 448 mg (14.5 mmol)/day.
Risk Characterization
Phosphorus exposure data indicate that only a small percentage of the U.S. population is likely to exceed the UL. Because phosphorus supplements are not widely consumed, nor is the dosage high, total intake from diet plus supplements would infrequently exceed the UL.
RESEARCH RECOMMENDATIONS
-
The model that relates absorbed phosphorus intake to serum phosphorus must be evaluated in clinical studies using oral phosphorus intakes, and investigated in children and adolescents as well as adults.
-
Bone mineral mass as a function of dietary phosphorus intake should be investigated at all stages of the life cycle.
-
The practical effect of phosphate-containing food additives on trace mineral status (iron, copper, and zinc) should be evaluated.












































